9.3: Model of Conduction in Metals
( \newcommand{\kernel}{\mathrm{null}\,}\)
By the end of this section, you will be able to:
- Define the drift velocity of charges moving through a metal
- Define the vector current density
- Describe the operation of an incandescent lamp
When electrons move through a conducting wire, they do not move at a constant velocity, that is, the electrons do not move in a straight line at a constant speed. Rather, they interact with and collide with atoms and other free electrons in the conductor. Thus, the electrons move in a zig-zag fashion and drift through the wire. We should also note that even though it is convenient to discuss the direction of current, current is a scalar quantity. When discussing the velocity of charges in a current, it is more appropriate to discuss the current density. We will come back to this idea at the end of this section.
Drift Velocity
Electrical signals move very rapidly. Telephone conversations carried by currents in wires cover large distances without noticeable delays. Lights come on as soon as a light switch is moved to the ‘on’ position. Most electrical signals carried by currents travel at speeds on the order of 108m/s a significant fraction of the speed of light. Interestingly, the individual charges that make up the current move much slower on average, typically drifting at speeds on the order of 10−4m/s. How do we reconcile these two speeds, and what does it tell us about standard conductors?
The high speed of electrical signals results from the fact that the force between charges acts rapidly at a distance. Thus, when a free charge is forced into a wire, as in Figure 9.3.1, the incoming charge pushes other charges ahead of it due to the repulsive force between like charges. These moving charges push on charges farther down the line. The density of charge in a system cannot easily be increased, so the signal is passed on rapidly. The resulting electrical shock wave moves through the system at nearly the speed of light. To be precise, this fast-moving signal, or shock wave, is a rapidly propagating change in the electrical field.

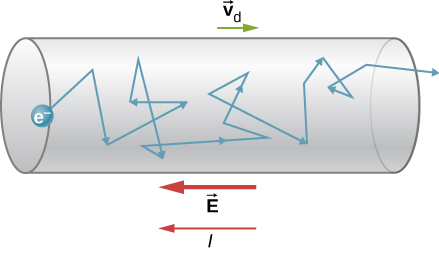
Good conductors have large numbers of free charges. In metals, the free charges are free electrons. (In fact, good electrical conductors are often good heat conductors too, because large numbers of free electrons can transport thermal energy as well as carry electrical current.) Figure 9.3.2 shows how free electrons move through an ordinary conductor. The distance that an individual electron can move between collisions with atoms or other electrons is quite small. The electron paths thus appear nearly random, like the motion of atoms in a gas. But there is an electrical field in the conductor that causes the electrons to drift in the direction shown (opposite to the field, since they are negative). The drift velocity →vd is the average velocity of the free charges. Drift velocity is quite small, since there are so many free charges. If we have an estimate of the density of free electrons in a conductor, we can calculate the drift velocity for a given current. The larger the density, the lower the velocity required for a given current.
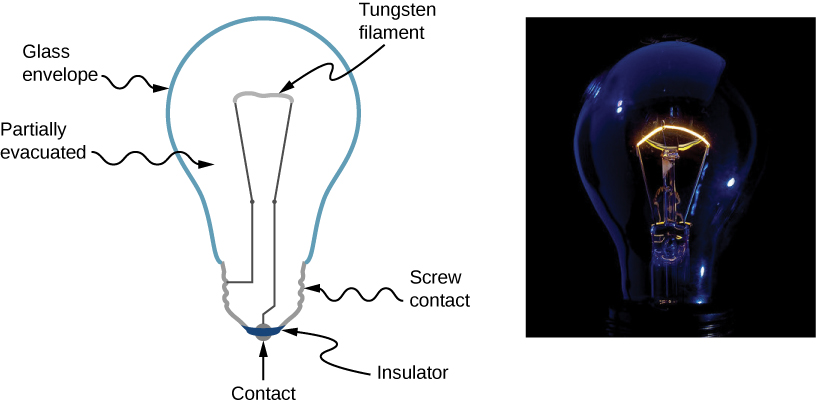
Free-electron collisions transfer energy to the atoms of the conductor. The electrical field does work in moving the electrons through a distance, but that work does not increase the kinetic energy (nor speed) of the electrons. The work is transferred to the conductor’s atoms, often increasing temperature. Thus, a continuous power input is required to keep a current flowing. (An exception is superconductors, for reasons we shall explore in a later chapter. Superconductors can have a steady current without a continual supply of energy—a great energy savings.) For a conductor that is not a superconductor, the supply of energy can be useful, as in an incandescent light bulb filament (Figure 9.3.3). The supply of energy is necessary to increase the temperature of the tungsten filament, so that the filament glows.
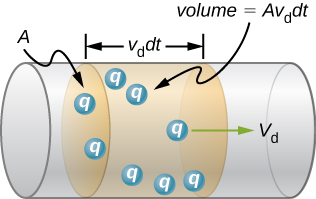
We can obtain an expression for the relationship between current and drift velocity by considering the number of free charges in a segment of wire, as illustrated in Figure 9.3.4. The number of free charges per unit volume, or the number density of free charges, is given the symbol n where
n=number of chargesvolume.
The value of n depends on the material. The shaded segment has a volume Avddt, so that the number of free charges in the volume is nAvddt. The charge dQ in this segment is thus qnAvddt, where q is the amount of charge on each carrier. (The magnitude of the charge of electrons is q=1.60×10−19C.) Current is charge moved per unit time; thus, if all the original charges move out of this segment in time dt, the current is
I=dQdt=qnAvd.
Rearranging terms gives
vd=InqA
where vd is the drift velocity, n is the free charge density, A is the cross-sectional area of the wire, and I is the current through the wire.
The carriers of the current each have charge q and move with a drift velocity of magnitude vd.
Note that simple drift velocity is not the entire story. The speed of an electron is sometimes much greater than its drift velocity. In addition, not all of the electrons in a conductor can move freely, and those that do move might move somewhat faster or slower than the drift velocity. So what do we mean by free electrons?
Atoms in a metallic conductor are packed in the form of a lattice structure. Some electrons are far enough away from the atomic nuclei that they do not experience the attraction of the nuclei as strongly as the inner electrons do. These are the free electrons. They are not bound to a single atom but can instead move freely among the atoms in a “sea” of electrons. When an electrical field is applied, these free electrons respond by accelerating. As they move, they collide with the atoms in the lattice and with other electrons, generating thermal energy, and the conductor gets warmer. In an insulator, the organization of the atoms and the structure do not allow for such free electrons.
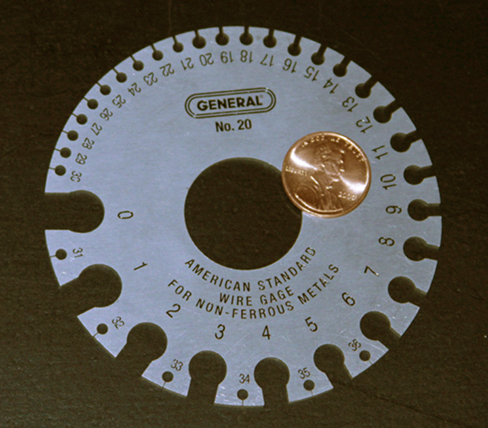
As you know, electric power is usually supplied to equipment and appliances through round wires made of a conducting material (copper, aluminum, silver, or gold) that are stranded or solid. The diameter of the wire determines the current-carrying capacity—the larger the diameter, the greater the current-carrying capacity. Even though the current-carrying capacity is determined by the diameter, wire is not normally characterized by the diameter directly. Instead, wire is commonly sold in a unit known as “gauge.” Wires are manufactured by passing the material through circular forms called “drawing dies.” In order to make thinner wires, manufacturers draw the wires through multiple dies of successively thinner diameter. Historically, the gauge of the wire was related to the number of drawing processes required to manufacture the wire. For this reason, the larger the gauge, the smaller the diameter. In the United States, the American Wire Gauge (AWG) was developed to standardize the system. Household wiring commonly consists of 10-gauge (2.588-mm diameter) to 14-gauge (1.628-mm diameter) wire. A device used to measure the gauge of wire is shown in Figure 9.3.5.
Calculate the drift velocity of electrons in a copper wire with a diameter of 2.053 mm (12-gauge) carrying a 20.0-A current, given that there is one free electron per copper atom. (Household wiring often contains 12-gauge copper wire, and the maximum current allowed in such wire is usually 20.0 A.) The density of copper is 8.80×103kg/m3 and the atomic mass of copper is 63.54 g/mol.
Strategy
We can calculate the drift velocity using the equation I=nqAvd. The current is I=20.00A and q=1.60×10−19C is the charge of an electron. We can calculate the area of a cross-section of the wire using the formula A=πr2, where r is one-half the diameter. The given diameter is 2.053 mm, so r is 1.0265 mm. We are given the density of copper, 8.80×103kg/m3, and the atomic mass of copper is 63.54g/mol. We can use these two quantities along with Avogadro’s number, 6.02×1023atoms/mol, to determine n, the number of free electrons per cubic meter.
Solution
First, we calculate the density of free electrons in copper. There is one free electron per copper atom. Therefore, the number of free electrons is the same as the number of copper atoms per m3. We can now find n as follows:
n=1e−atom×6.02×1023atomsmol×1mol63.54g×1000gkg×8.80×103kg1m3=8.34×1028e−/m3.
The cross-sectional area of the wire is
A=πr2=π(2.05×10−3m2)2=3.30×10−6m2.
Rearranging I=nqAvd to isolate drift velocity gives
vd=InqA=20.00A(8.34×1028/m3)(−1.60×10−19C)(3.30×10−6m2)=−4.54×10−4m/s.
Significance
The minus sign indicates that the negative charges are moving in the direction opposite to conventional current. The small value for drift velocity (on the order of 10−4m/s confirms that the signal moves on the order of 1012 times faster (about 108m/s than the charges that carry it.
In Example 9.3.1, the drift velocity was calculated for a 2.053-mm diameter (12-gauge) copper wire carrying a 20-amp current. Would the drift velocity change for a 1.628-mm diameter (14-gauge) wire carrying the same 20-amp current?
- Answer
-
The diameter of the 14-gauge wire is smaller than the diameter of the 12-gauge wire. Since the drift velocity is inversely proportional to the cross-sectional area, the drift velocity in the 14-gauge wire is larger than the drift velocity in the 12-gauge wire carrying the same current. The number of electrons per cubic meter will remain constant.
Current Density
Although it is often convenient to attach a negative or positive sign to indicate the overall direction of motion of the charges, current is a scalar quantity, I=dQdt. It is often necessary to discuss the details of the motion of the charge, instead of discussing the overall motion of the charges. In such cases, it is necessary to discuss the current density, →J, a vector quantity. The current density is the flow of charge through an infinitesimal area, divided by the area. The current density must take into account the local magnitude and direction of the charge flow, which varies from point to point. The unit of current density is ampere per meter squared, and the direction is defined as the direction of net flow of positive charges through the area.
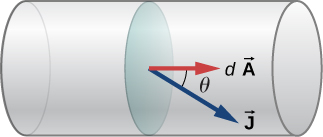
The relationship between the current and the current density can be seen in Figure 9.3.6. The differential current flow through the area d→A is found as
dI=→J⋅d→A=JdAcosθ,
where θ is the angle between the area and the current density. The total current passing through area d→A can be found by integrating over the area,
I=∬area→J⋅d→A.
Consider the magnitude of the current density, which is the current divided by the area:
J=IA=n|q|AvdA=n|q|vd.
Thus, the current density is →J=nq→vd. If q is positive, →vd is in the same direction as the electrical field →E. If q is negative, →vd is in the opposite direction of →E. Either way, the direction of the current density →J is in the direction of the electrical field →E.
The current supplied to a lamp with a 100-W light bulb is 0.87 amps. The lamp is wired using a copper wire with diameter 2.588 mm (10-gauge). Find the magnitude of the current density.
Strategy
The current density is the current moving through an infinitesimal cross-sectional area divided by the area. We can calculate the magnitude of the current density using J=IA. The current is given as 0.87 A. The cross-sectional area can be calculated to be A=5.26mm2.
Solution
Calculate the current density using the given current I=0.87A and the area, found to be A=5.26mm2.
J=IA=0.87A5.26×10−6m2=1.65×105Am2.
Significance
The current density in a conducting wire depends on the current through the conducting wire and the cross-sectional area of the wire. For a given current, as the diameter of the wire increases, the charge density decreases.
The current density is proportional to the current and inversely proportional to the area. If the current density in a conducting wire increases, what would happen to the drift velocity of the charges in the wire?
- Answer
-
The current density in a conducting wire increases due to an increase in current. The drift velocity is inversely proportional to the current (vd=nqAI), so the drift velocity would decrease.
What is the significance of the current density? The current density is proportional to the current, and the current is the number of charges that pass through a cross-sectional area per second. The charges move through the conductor, accelerated by the electric force provided by the electrical field. The electrical field is created when a voltage is applied across the conductor. In Ohm’s Law, we will use this relationship between the current density and the electrical field to examine the relationship between the current through a conductor and the voltage applied.


