11.3: Magnetic Fields and Lines
( \newcommand{\kernel}{\mathrm{null}\,}\)
By the end of this section, you will be able to:
- Define the magnetic field based on a moving charge experiencing a force
- Apply the right-hand rule to determine the direction of a magnetic force based on the motion of a charge in a magnetic field
- Sketch magnetic field lines to understand which way the magnetic field points and how strong it is in a region of space
We have outlined the properties of magnets, described how they behave, and listed some of the applications of magnetic properties. Even though there are no such things as isolated magnetic charges, we can still define the attraction and repulsion of magnets as based on a field. In this section, we define the magnetic field, determine its direction based on the right-hand rule, and discuss how to draw magnetic field lines.
Defining the Magnetic Field
A magnetic field is defined by the force that a charged particle experiences moving in this field, after we account for the gravitational and any additional electric forces possible on the charge. The magnitude of this force is proportional to the amount of charge q, the speed of the charged particle v, and the magnitude of the applied magnetic field. The direction of this force is perpendicular to both the direction of the moving charged particle and the direction of the applied magnetic field. Based on these observations, we define the magnetic field strength B based on the magnetic force →F on a charge q moving at velocity as the cross product of the velocity and magnetic field, that is,
→F=q→v×→B.
In fact, this is how we define the magnetic field →B - in terms of the force on a charged particle moving in a magnetic field. The magnitude of the force is determined from the definition of the cross product as it relates to the magnitudes of each of the vectors. In other words, the magnitude of the force satisfies
F=qvBsinθ
where θ is the angle between the velocity and the magnetic field.
The SI unit for magnetic field strength B is called the tesla (T) after the eccentric, but brilliant inventor Nikola Tesla (1856–1943), where
1T=1NA⋅m.
A smaller unit, called the gauss (G) is sometimes used, where
1G=10−4T
The strongest permanent magnets have fields near 2 T; superconducting electromagnets may attain 10 T or more. Earth’s magnetic field on its surface is only about 5×10−5T or 0.5G.
The direction of the magnetic force →F is perpendicular to the plane formed by →v and →B as determined by the right-hand rule-1 (or RHR-1), which is illustrated in Figure 11.3.1.
- Orient your right hand so that your fingers curl in the plane defined by the velocity and magnetic field vectors.
- Using your right hand, sweep from the velocity toward the magnetic field with your fingers through the smallest angle possible.
- The magnetic force is directed where your thumb is pointing.
- If the charge was negative, reverse the direction found by these steps.
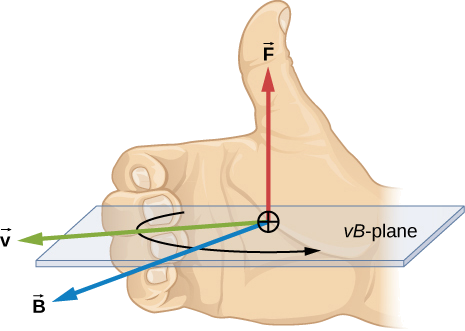
Figure 11.3.1: Magnetic fields exert forces on moving charges. The direction of the magnetic force on a moving charge is perpendicular to the plane formed by b→v and →B and follows the right-hand rule-1 (RHR-1) as shown. The magnitude of the force is proportional to q,v,B, and the sine of the angle between →v and →B.
Visit this website for additional practice with the direction of magnetic fields.
There is no magnetic force on static charges. However, there is a magnetic force on charges moving at an angle to a magnetic field. When charges are stationary, their electric fields do not affect magnets. However, when charges move, they produce magnetic fields that exert forces on other magnets. When there is relative motion, a connection between electric and magnetic forces emerges - each affects the other.
An alpha-particle (q=3.2×10−19C) moves through a uniform magnetic field whose magnitude is 1.5 T. The field is directly parallel to the positive z-axis of the rectangular coordinate system of Figure 11.3.2. What is the magnetic force on the alpha-particle when it is moving (a) in the positive x-direction with a speed of 5.0×104m/s? (b) in the negative y-direction with a speed of 5.0×104m/s? (c) in the positive z-direction with a speed of 5.0×104m/s? (d) with a velocity →v=(2.0ˆi−3.0ˆj+1.0ˆk)×104m/s?
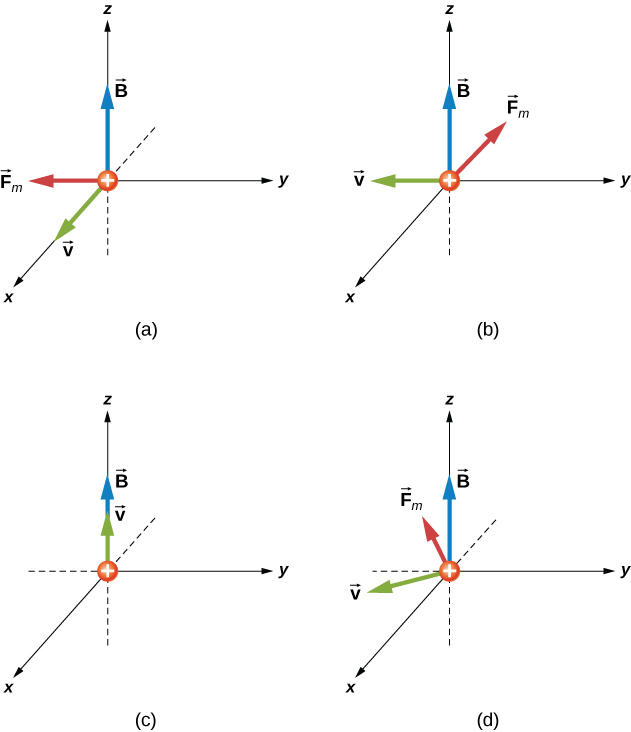
Strategy
We are given the charge, its velocity, and the magnetic field strength and direction. We can thus use the equation →F=q→v×→B or F=qvBsinθ to calculate the force. The direction of the force is determined by RHR-1.
Solution
- First, to determine the direction, start with your fingers pointing in the positive x-direction. Sweep your fingers upward in the direction of magnetic field. Your thumb should point in the negative y-direction. This should match the mathematical answer. To calculate the force, we use the given charge, velocity, and magnetic field and the definition of the magnetic force in cross-product form to calculate: →F=q→v×→B=(3.2×10−19C)(5.0×104m/sˆi)×(1.5Tˆk)=−2.4×10−14Nˆj
- First, to determine the directionality, start with your fingers pointing in the negative y-direction. Sweep your fingers upward in the direction of magnetic field as in the previous problem. Your thumb should be open in the negative x-direction. This should match the mathematical answer. To calculate the force, we use the given charge, velocity, and magnetic field and the definition of the magnetic force in cross-product form to calculate: →F=q→v×→B=(3.2×10−19C)(−5.0×104m/sˆi)×(1.5Tˆk)=−2.4×10−14Nˆi An alternative approach is to use Equation ??? to find the magnitude of the force. This applies for both parts (a) and (b). Since the velocity is perpendicular to the magnetic field, the angle between them is 90 degrees. Therefore, the magnitude of the force is: F=qvBsinθ=(3.2×10−19C)(5.0×104m/s)(1.5T)sin(90o))=2.4×10−14N.
- Since the velocity and magnetic field are parallel to each other, there is no orientation of your hand that will result in a force direction. Therefore, the force on this moving charge is zero. This is confirmed by the cross product. When you cross two vectors pointing in the same direction, the result is equal to zero.
- First, to determine the direction, your fingers could point in any orientation; however, you must sweep your fingers upward in the direction of the magnetic field. As you rotate your hand, notice that the thumb can point in any x- or y-direction possible, but not in the z-direction. This should match the mathematical answer. To calculate the force, we use the given charge, velocity, and magnetic field and the definition of the magnetic force in cross-product form to calculate: →F=q→v×→B=(3.2×10−19C)((2.0ˆi−3.0ˆj+1.0ˆk)×104m/s)×(1.5Tˆk) (−14.4ˆi−9.6ˆj)×10−15N. This solution can be rewritten in terms of a magnitude and angle in the xy-plane: |→F|=√F2x+F2y=√(−14.4)2+(−9.6)2×10−15N=1.7×10−14N θ=tan−1(FyFx)=tan−1(−9.6×10−15N−14.4×10−15N)=34o. The magnitude of the force can also be calculated using Equation ???. The velocity in this question, however, has three components. The z-component of the velocity can be neglected, because it is parallel to the magnetic field and therefore generates no force. The magnitude of the velocity is calculated from the x- and y-components. The angle between the velocity in the xy-plane and the magnetic field in the z-plane is 90 degrees. Therefore, the force is calculated to be: |→v|=√(2)2+(−3)2×104ms=3.6×104msF=qvBsinθ=(3.2×10−19C)(3.6×104m/s)(1.5T)sin(90o)=1.7×10−14N
Significance
The cross product in this formula results in a third vector that must be perpendicular to the other two. Other physical quantities, such as angular momentum, also have three vectors that are related by the cross product. Note that typical force values in magnetic force problems are much larger than the gravitational force. Therefore, for an isolated charge, the magnetic force is the dominant force governing the charge’s motion.
Repeat the previous problem with the magnetic field in the x-direction rather than in the z-direction. Check your answers with RHR-1.
- Answer a
-
0 N
- Answer b
-
2.4×10−14ˆkN
- Answer c
-
2.4×10−14ˆjN
- Answer d
-
7.2ˆj+2.2ˆk)×10−15N
Representing Magnetic Fields
The representation of magnetic fields by magnetic field lines is very useful in visualizing the strength and direction of the magnetic field. As shown in Figure 11.3.3, each of these lines forms a closed loop, even if not shown by the constraints of the space available for the figure. The field lines emerge from the north pole (N), loop around to the south pole (S), and continue through the bar magnet back to the north pole.
Magnetic field lines have several hard-and-fast rules:
- The direction of the magnetic field is tangent to the field line at any point in space. A small compass will point in the direction of the field line.
- The strength of the field is proportional to the closeness of the lines. It is exactly proportional to the number of lines per unit area perpendicular to the lines (called the areal density).
- Magnetic field lines can never cross, meaning that the field is unique at any point in space.
- Magnetic field lines are continuous, forming closed loops without a beginning or end. They are directed from the north pole to the south pole.
The last property is related to the fact that the north and south poles cannot be separated. It is a distinct difference from electric field lines, which generally begin on positive charges and end on negative charges or at infinity. If isolated magnetic charges (referred to as magnetic monopoles) existed, then magnetic field lines would begin and end on them.



