22.E: Magnetism (Exercises)

Conceptual Questions

22.1: Magnets

1. Volcanic and other such activity at the mid-Atlantic ridge extrudes material to fill the gap between separating tectonic plates associated with continental drift. The magnetization of rocks is found to reverse in a coordinated manner with distance from the ridge. What does this imply about the Earth’s magnetic field and how could the knowledge of the spreading rate be used to give its historical record?

22.3: Magnetic Fields and Magnetic Field Lines

2. Explain why the magnetic field would not be unique (that is, not have a single value) at a point in space where magnetic field lines might cross. (Consider the direction of the field at such a point.)

3. List the ways in which magnetic field lines and electric field lines are similar. For example, the field direction is tangent to the line at any point in space. Also list the ways in which they differ. For example, electric force is parallel to electric field lines, whereas magnetic force on moving charges is perpendicular to magnetic field lines.

4. Noting that the magnetic field lines of a bar magnet resemble the electric field lines of a pair of equal and opposite charges, do you expect the magnetic field to rapidly decrease in strength with distance from the magnet? Is this consistent with your experience with magnets?

5. Is the Earth’s magnetic field parallel to the ground at all locations? If not, where is it parallel to the surface? Is its strength the same at all locations? If not, where is it greatest?

6. If a charged particle moves in a straight line through some region of space, can you say that the magnetic field in that region is necessarily zero?

22.4: Magnetic Field Strength: Force on a Moving Charge in a Magnetic Field

7. If a charged particle moves in a straight line through some region of space, can you say that the magnetic field in that region is necessarily zero?

22.5: Force on a Moving Charge in a Magnetic Field: Examples and Applications

8. How can the motion of a charged particle be used to distinguish between a magnetic and an electric field?

9. High-velocity charged particles can damage biological cells and are a component of radiation exposure in a variety of locations ranging from research facilities to natural background. Describe how you could use a magnetic field to shield yourself.

10. If a cosmic ray proton approaches the Earth from outer space along a line toward the center of the Earth that lies in the plane of the equator, in what direction will it be deflected by the Earth’s magnetic field? What about an electron? A neutron?

11. What are the signs of the charges on the particles in Figure?

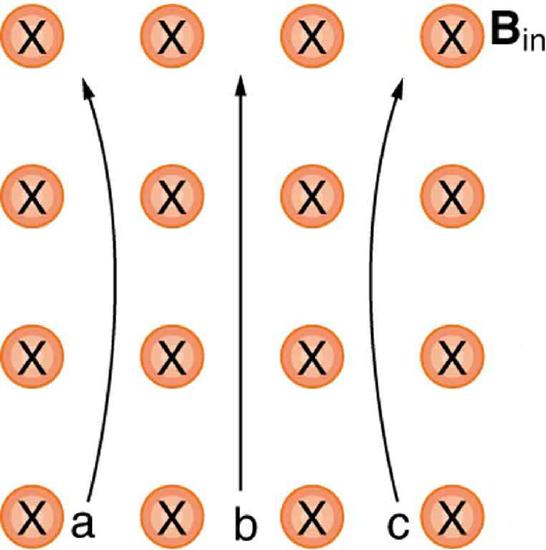
12. Which of the particles in Figure has the greatest velocity, assuming they have identical charges and masses?

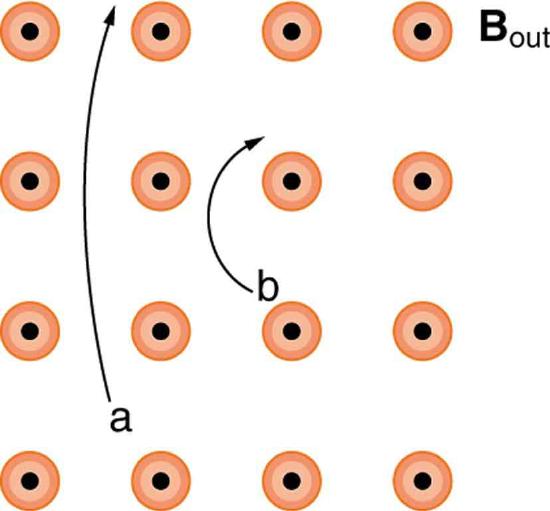
13. Which of the particles in Figure has the greatest mass, assuming all have identical charges and velocities?

14. While operating, a high-precision TV monitor is placed on its side during maintenance. The image on the monitor changes color and blurs slightly. Discuss the possible relation of these effects to the Earth’s magnetic field.

22.6: The Hall Effect

15. Discuss how the Hall effect could be used to obtain information on free charge density in a conductor. (Hint: Consider how drift velocity and current are related.)

22.7: Magnetic Force on a Current-Carrying Conductor

16. Draw a sketch of the situation in Figure showing the direction of electrons carrying the current, and use RHR-1 to verify the direction of the force on the wire.

17. Verify that the direction of the force in an MHD drive, such as that in Figure, does not depend on the sign of the charges carrying the current across the fluid.

18. Why would a magnetohydrodynamic drive work better in ocean water than in fresh water? Also, why would superconducting magnets be desirable?

19. Which is more likely to interfere with compass readings, AC current in your refrigerator or DC current when you start your car? Explain.

22.8: Torque on a Current Loop: Motors and Meters

20. Draw a diagram and use RHR-1 to show that the forces on the top and bottom segments of the motor’s current loop in Figure are vertical and produce no torque about the axis of rotation.

22.9: Magnetic Fields Produced by Currents: Ampere’s Law

21. Make a drawing and use RHR-2 to find the direction of the magnetic field of a current loop in a motor (such as in [link]). Then show that the direction of the torque on the loop is the same as produced by like poles repelling and unlike poles attracting.

22.10: Magnetic Force between Two Parallel Conductors

22. Is the force attractive or repulsive between the hot and neutral lines hung from power poles? Why?

23. If you have three parallel wires in the same plane, as in Figure, with currents in the outer two running in opposite directions, is it possible for the middle wire to be repelled by both? Attracted by both? Explain.

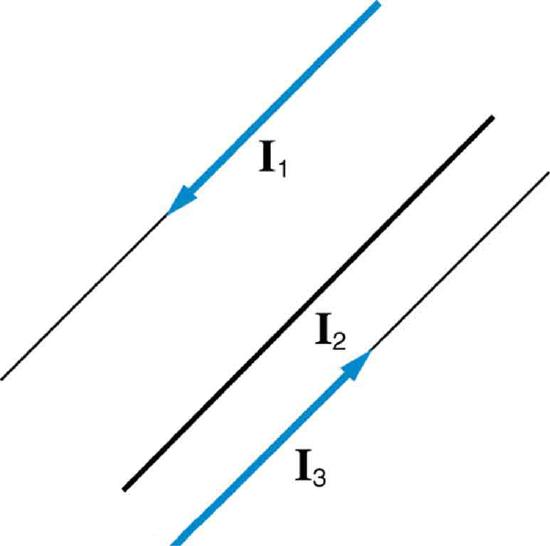
Three parallel coplanar wires with currents in the outer two in opposite directions.

24. Suppose two long straight wires run perpendicular to one another without touching. Does one exert a net force on the other? If so, what is its direction? Does one exert a net torque on the other? If so, what is its direction? Justify your responses by using the right hand rules.

25. Use the right hand rules to show that the force between the two loops in Figure is attractive if the currents are in the same direction and repulsive if they are in opposite directions. Is this consistent with like poles of the loops repelling and unlike poles of the loops attracting? Draw sketches to justify your answers.

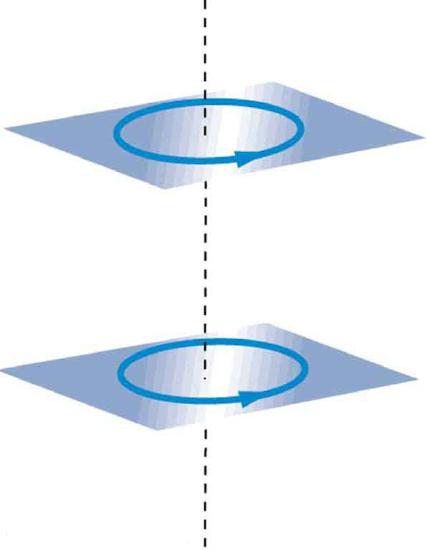
Two loops of wire carrying currents can exert forces and torques on one another.

26. f one of the loops in Figure is tilted slightly relative to the other and their currents are in the same direction, what are the directions of the torques they exert on each other? Does this imply that the poles of the bar magnet-like fields they create will line up with each other if the loops are allowed to rotate?

27. Electric field lines can be shielded by the Faraday cage effect. Can we have magnetic shielding? Can we have gravitational shielding?

22.11 More Applications of Magnetism

28. Measurements of the weak and fluctuating magnetic fields associated with brain activity are called magnetoencephalograms (MEGs). Do the brain’s magnetic fields imply coordinated or uncoordinated nerve impulses? Explain.

29. Discuss the possibility that a Hall voltage would be generated on the moving heart of a patient during MRI imaging. Also discuss the same effect on the wires of a pacemaker. (The fact that patients with pacemakers are not given MRIs is significant.)

30. A patient in an MRI unit turns his head quickly to one side and experiences momentary dizziness and a strange taste in his mouth. Discuss the possible causes.

31. You are told that in a certain region there is either a uniform electric or magnetic field. What measurement or observation could you make to determine the type? (Ignore the Earth’s magnetic field.)

32. An example of magnetohydrodynamics (MHD) comes from the flow of a river (salty water). This fluid interacts with the Earth’s magnetic field to produce a potential difference between the two river banks. How would you go about calculating the potential difference?

33. Draw gravitational field lines between 2 masses, electric field lines between a positive and a negative charge, electric field lines between 2 positive charges and magnetic field lines around a magnet. Qualitatively describe the differences between the fields and the entities responsible for the field lines.

Problems & Exercises

22.4: Magnetic Field Strength: Force on a Moving Charge in a Magnetic Field

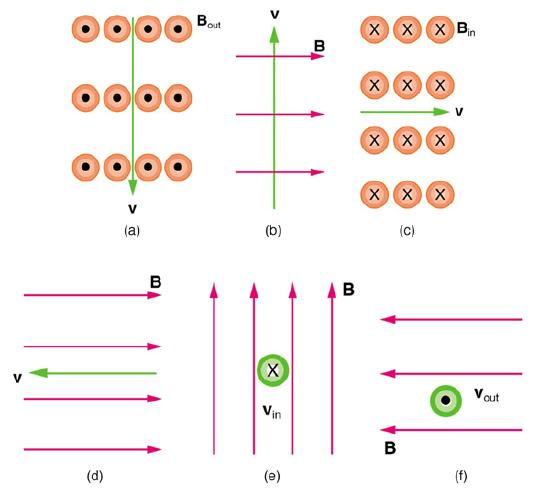
34. What is the direction of the magnetic force on a positive charge that moves as shown in each of the six cases shown in Figure?

Solution
(a) Left (West)
(b) Into the page
(c) Up (North)
(d) No force
(e) Right (East)
(f) Down (South)

35. Repeat Exercise 34 for a negative charge.

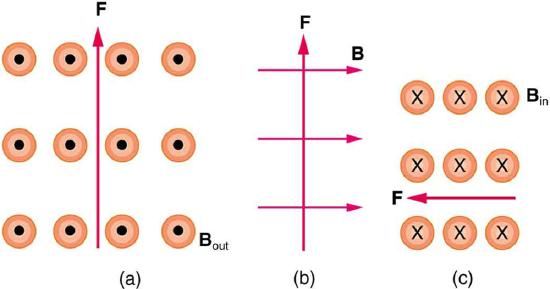
36. What is the direction of the velocity of a negative charge that experiences the magnetic force shown in each of the three cases in Figure, assuming it moves perpendicular to \(\displaystyle B\)?

Solution
(a) East (right)
(b) Into page
(c) South (down)

37. Repeat Exercise 36 for a positive charge.

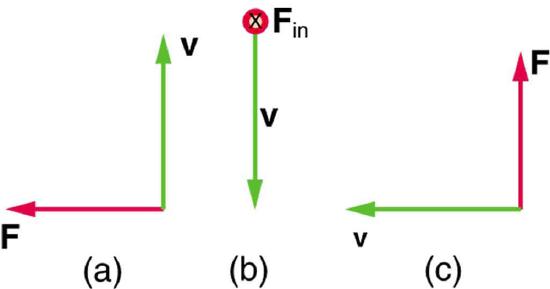
38. What is the direction of the magnetic field that produces the magnetic force on a positive charge as shown in each of the three cases in the figure below, assuming \(\displaystyle B\) is perpendicular to \(\displaystyle v\)?

Solution
(a) Into page
(b) West (left)
(c) Out of page

39. Repeat Exercise 38 for a negative charge.

40. What is the maximum force on an aluminum rod with a \(\displaystyle 0.100-μC\) charge that you pass between the poles of a 1.50-T permanent magnet at a speed of 5.00 m/s? In what direction is the force?

Solution
\(\displaystyle 7.50×10^{−7}N\) perpendicular to both the magnetic field lines and the velocity

41. (a) Aircraft sometimes acquire small static charges. Suppose a supersonic jet has a \(\displaystyle 0.500-μC\) charge and flies due west at a speed of 660 m/s over the Earth’s south magnetic pole, where the \(\displaystyle 8.00×10^{−5}-T\) magnetic field points straight up. What are the direction and the magnitude of the magnetic force on the plane?

(b) Discuss whether the value obtained in part (a) implies this is a significant or negligible effect.

42. (a) A cosmic ray proton moving toward the Earth at \(\displaystyle 5.00×107^m/s\) experiences a magnetic force of \(\displaystyle 1.70×10^{−16}N\). What is the strength of the magnetic field if there is a \(\displaystyle 45º\) angle between it and the proton’s velocity?

(b) Is the value obtained in part (a) consistent with the known strength of the Earth’s magnetic field on its surface? Discuss.

Solution
(a) \(\displaystyle 3.01×10^{−5}T\)
(b) This is slightly less then the magnetic field strength of \(\displaystyle 5×10^{−5}T\) at the surface of the Earth, so it is consistent.

43. An electron moving at \(\displaystyle 4.00×10^3m/s\) in a 1.25-T magnetic field experiences a magnetic force of \(\displaystyle 1.40×10^{−16}N\). What angle does the velocity of the electron make with the magnetic field? There are two answers.

44. (a) A physicist performing a sensitive measurement wants to limit the magnetic force on a moving charge in her equipment to less than \(\displaystyle 1.00×10^{−12}N\). What is the greatest the charge can be if it moves at a maximum speed of 30.0 m/s in the Earth’s field?

(b) Discuss whether it would be difficult to limit the charge to less than the value found in (a) by comparing it with typical static electricity and noting that static is often absent.

Solution
(a) \(\displaystyle 6.67×10^{−10}C\) (taking the Earth’s field to be \(\displaystyle 5.00×10^{−5}T\))
(b) Less than typical static, therefore difficult

22.5: Force on a Moving Charge in a Magnetic Field: Examples and Applications

45. A cosmic ray electron moves at \(\displaystyle 7.50×10^6m/s\) perpendicular to the Earth’s magnetic field at an altitude where field strength is \(\displaystyle 1.00×10^{−5}T\). What is the radius of the circular path the electron follows?

Solution
4.27 m

46. A proton moves at \(\displaystyle 7.50×10^7m/s\) perpendicular to a magnetic field. The field causes the proton to travel in a circular path of radius 0.800 m. What is the field strength?

47. (a) Viewers of Star Trek hear of an antimatter drive on the Starship Enterprise. One possibility for such a futuristic energy source is to store antimatter charged particles in a vacuum chamber, circulating in a magnetic field, and then extract them as needed. Antimatter annihilates with normal matter, producing pure energy. What strength magnetic field is needed to hold antiprotons, moving at \(\displaystyle 5.00×10^7m/s\) in a circular path 2.00 m in radius? Antiprotons have the same mass as protons but the opposite (negative) charge.

(b) Is this field strength obtainable with today’s technology or is it a futuristic possibility?

Solution
(a) 0.261 T
(b) This strength is definitely obtainable with today’s technology. Magnetic field strengths of 0.500 T are obtainable with permanent magnets.

48. (a) An oxygen-16 ion with a mass of \(\displaystyle 2.66×10^{−26}kg\) travels at \(\displaystyle 5.00×10^6\) perpendicular to a 1.20-T magnetic field, which makes it move in a circular arc with a 0.231-m radius. What positive charge is on the ion?

(b) What is the ratio of this charge to the charge of an electron?

(c) Discuss why the ratio found in (b) should be an integer.

49. What radius circular path does an electron travel if it moves at the same speed and in the same magnetic field as the proton in Exercise?

Solution
\(\displaystyle 4.36×10^{−4}m\)

50. A velocity selector in a mass spectrometer uses a 0.100-T magnetic field.

(a) What electric field strength is needed to select a speed of \(\displaystyle 4.00×10^6\)m/s?

(b) What is the voltage between the plates if they are separated by 1.00 cm?

51. An electron in a TV CRT moves with a speed of \(\displaystyle 6.00×10^7m/s\), in a direction perpendicular to the Earth’s field, which has a strength of \(\displaystyle 5.00×10^{−5}T\).

(a) What strength electric field must be applied perpendicular to the Earth’s field to make the electron moves in a straight line?

(b) If this is done between plates separated by 1.00 cm, what is the voltage applied? (Note that TVs are usually surrounded by a ferromagnetic material to shield against external magnetic fields and avoid the need for such a correction.)

Solution
(a) 3.00 kV/m
(b) 30.0 V

52. (a) At what speed will a proton move in a circular path of the same radius as the electron in Exercise?

(b) What would the radius of the path be if the proton had the same speed as the electron?

(c) What would the radius be if the proton had the same kinetic energy as the electron?

(d) The same momentum?

53. A mass spectrometer is being used to separate common oxygen-16 from the much rarer oxygen-18, taken from a sample of old glacial ice. (The relative abundance of these oxygen isotopes is related to climatic temperature at the time the ice was deposited.) The ratio of the masses of these two ions is 16 to 18, the mass of oxygen-16 is \(\displaystyle 2.66×10^{−26}kg\), and they are singly charged and travel at \(\displaystyle 5.00×10^6m/s\) in a 1.20-T magnetic field. What is the separation between their paths when they hit a target after traversing a semicircle?

Solution
0.173 m

54. (a) Triply charged uranium-235 and uranium-238 ions are being separated in a mass spectrometer. (The much rarer uranium-235 is used as reactor fuel.) The masses of the ions are \(\displaystyle 3.90×10^{−25}kg\) and \(\displaystyle 3.95×10^{−25}kg\), respectively, and they travel at \(\displaystyle 3.00×10^5m/s\) in a 0.250-T field. What is the separation between their paths when they hit a target after traversing a semicircle? (b) Discuss whether this distance between their paths seems to be big enough to be practical in the separation of uranium-235 from uranium-238.

22.6: The Hall Effect

55. A large water main is 2.50 m in diameter and the average water velocity is 6.00 m/s. Find the Hall voltage produced if the pipe runs perpendicular to the Earth’s \(\displaystyle 5.00×10^{−5}-T\) field.

Solution
\(\displaystyle 7.50×10^{−4}V\)

56. What Hall voltage is produced by a 0.200-T field applied across a 2.60-cm-diameter aorta when blood velocity is 60.0 cm/s?

57. (a) What is the speed of a supersonic aircraft with a 17.0-m wingspan, if it experiences a 1.60-V Hall voltage between its wing tips when in level flight over the north magnetic pole, where the Earth’s field strength is \(\displaystyle 8.00×10^{−5}T\)?

(b) Explain why very little current flows as a result of this Hall voltage.

Solution
(a) \(\displaystyle 1.18 × 10^3 m/s\)
(b) Once established, the Hall emf pushes charges one direction and the magnetic force acts in the opposite direction resulting in no net force on the charges. Therefore, no current flows in the direction of the Hall emf. This is the same as in a current-carrying conductor—current does not flow in the direction of the Hall emf.

58. A nonmechanical water meter could utilize the Hall effect by applying a magnetic field across a metal pipe and measuring the Hall voltage produced. What is the average fluid velocity in a 3.00-cm-diameter pipe, if a 0.500-T field across it creates a 60.0-mV Hall voltage?

59. Calculate the Hall voltage induced on a patient’s heart while being scanned by an MRI unit. Approximate the conducting path on the heart wall by a wire 7.50 cm long that moves at 10.0 cm/s perpendicular to a 1.50-T magnetic field.

Solution
11.3 mV

60. A Hall probe calibrated to read \(\displaystyle 1.00 μV\) when placed in a 2.00-T field is placed in a 0.150-T field. What is its output voltage?

61. Using information in [link], what would the Hall voltage be if a 2.00-T field is applied across a 10-gauge copper wire (2.588 mm in diameter) carrying a 20.0-A current?

Solution
\(\displaystyle 1.16 μV\)

62. Show that the Hall voltage across wires made of the same material, carrying identical currents, and subjected to the same magnetic field is inversely proportional to their diameters. (Hint: Consider how drift velocity depends on wire diameter.)

63. A patient with a pacemaker is mistakenly being scanned for an MRI image. A 10.0-cm-long section of pacemaker wire moves at a speed of 10.0 cm/s perpendicular to the MRI unit’s magnetic field and a 20.0-mV Hall voltage is induced. What is the magnetic field strength?

Solution
2.00 T

22.7: Magnetic Force on a Current-Carrying Conductor

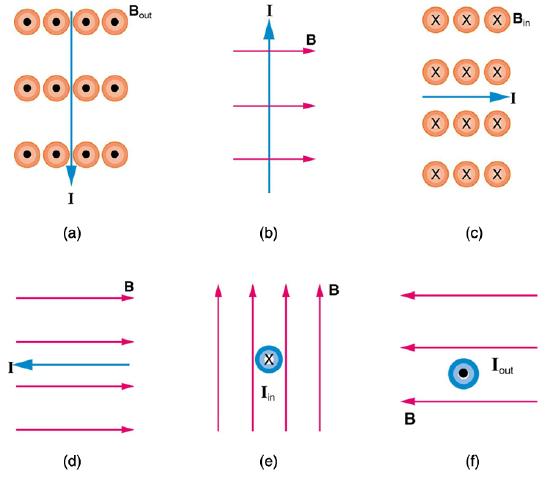
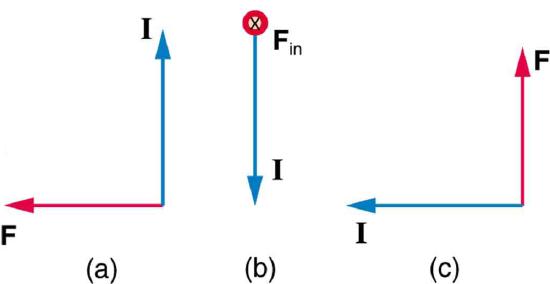
64. What is the direction of the magnetic force on the current in each of the six cases in Figure?

Solution
(a) west (left)
(b) into page
(c) north (up)
(d) no force
(e) east (right)
(f) south (down)

65. What is the direction of a current that experiences the magnetic force shown in each of the three cases in Figure, assuming the current runs perpendicular to \(\displaystyle B\)?

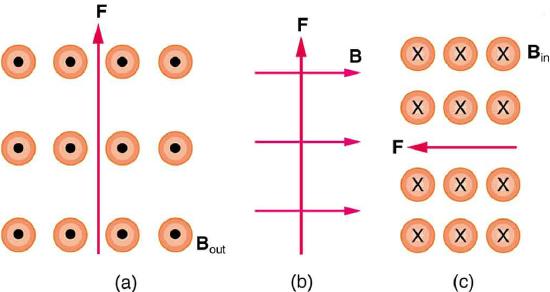
66. What is the direction of the magnetic field that produces the magnetic force shown on the currents in each of the three cases in Figure, assuming \(\displaystyle B\) is perpendicular to \(\displaystyle I\)?

Solution
(a) into page
(b) west (left)
(c) out of page

67. (a) What is the force per meter on a lightning bolt at the equator that carries 20,000 A perpendicular to the Earth’s \(\displaystyle 3.00×10^{−5}-T\) field?

(b) What is the direction of the force if the current is straight up and the Earth’s field direction is due north, parallel to the ground?

68. (a) A DC power line for a light-rail system carries 1000 A at an angle of \(\displaystyle 30.0º\) to the Earth’s \(\displaystyle 5.00×10^{−5}-T\) field. What is the force on a 100-m section of this line?

(b) Discuss practical concerns this presents, if any.

Solution
(a) 2.50 N
(b) This is about half a pound of force per 100 m of wire, which is much less than the weight of the wire itself. Therefore, it does not cause any special concerns.

69. What force is exerted on the water in an MHD drive utilizing a 25.0-cm-diameter tube, if 100-A current is passed across the tube that is perpendicular to a 2.00-T magnetic field? (The relatively small size of this force indicates the need for very large currents and magnetic fields to make practical MHD drives.)

70. A wire carrying a 30.0-A current passes between the poles of a strong magnet that is perpendicular to its field and experiences a 2.16-N force on the 4.00 cm of wire in the field. What is the average field strength?

Solution
1.80 T

71. (a) A 0.750-m-long section of cable carrying current to a car starter motor makes an angle of 60º with the Earth’s \(\displaystyle 5.50×10^{−5}T\) field. What is the current when the wire experiences a force of \(\displaystyle 7.00×10^{−3}N\)?

(b) If you run the wire between the poles of a strong horseshoe magnet, subjecting 5.00 cm of it to a 1.75-T field, what force is exerted on this segment of wire?

72. (a) What is the angle between a wire carrying an 8.00-A current and the 1.20-T field it is in if 50.0 cm of the wire experiences a magnetic force of 2.40 N? (b) What is the force on the wire if it is rotated to make an angle of \(\displaystyle 90º\) with the field?

Solution
(a) \(\displaystyle 30º\)
(b) 4.80 N

73. The force on the rectangular loop of wire in the magnetic field in Figure can be used to measure field strength. The field is uniform, and the plane of the loop is perpendicular to the field.

(a) What is the direction of the magnetic force on the loop? Justify the claim that the forces on the sides of the loop are equal and opposite, independent of how much of the loop is in the field and do not affect the net force on the loop.

(b) If a current of 5.00 A is used, what is the force per tesla on the 20.0-cm-wide loop?

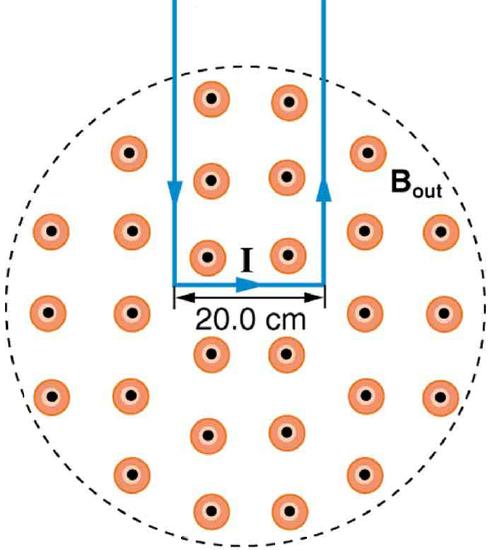
A rectangular loop of wire carrying a current is perpendicular to a magnetic field. The field is uniform in the region shown and is zero outside that region.

22.8: Torque on a Current Loop: Motors and Meters

74. (a) By how many percent is the torque of a motor decreased if its permanent magnets lose 5.0% of their strength?

(b) How many percent would the current need to be increased to return the torque to original values?

Solution
(a) \(\displaystyle τ\) decreases by 5.00% if B decreases by 5.00%
(b) 5.26% increase

75. (a) What is the maximum torque on a 150-turn square loop of wire 18.0 cm on a side that carries a 50.0-A current in a 1.60-T field?

(b) What is the torque when \(\displaystyle θ\) is \(\displaystyle 10.9º\)?

76. Find the current through a loop needed to create a maximum torque of \(\displaystyle 9.00 N⋅m\). The loop has 50 square turns that are 15.0 cm on a side and is in a uniform 0.800-T magnetic field.

Solution
10.0 A

77. Calculate the magnetic field strength needed on a 200-turn square loop 20.0 cm on a side to create a maximum torque of \(\displaystyle 300 N⋅m\) if the loop is carrying 25.0 A.

78. Since the equation for torque on a current-carrying loop is \(\displaystyle τ=NIABsinθ\), the units of \(\displaystyle N⋅m\) must equal units of \(\displaystyle A⋅m^2T\). Verify this.

Solution
\(\displaystyle A⋅m^2⋅T=A⋅m^2(\frac{N}{A⋅m})=N⋅m\)

79. (a) At what angle \(\displaystyle θ\) is the torque on a current loop 90.0% of maximum?

(b) 50.0% of maximum?

(c) 10.0% of maximum?

80. A proton has a magnetic field due to its spin on its axis. The field is similar to that created by a circular current loop \(\displaystyle 0.650×10^{−15}m\) in radius with a current of \(\displaystyle 1.05×10^4A\) (no kidding). Find the maximum torque on a proton in a 2.50-T field. (This is a significant torque on a small particle.)

Solution
\(\displaystyle 3.48×10^{−26}N⋅m\)

81. (a) A 200-turn circular loop of radius 50.0 cm is vertical, with its axis on an east-west line. A current of 100 A circulates clockwise in the loop when viewed from the east. The Earth’s field here is due north, parallel to the ground, with a strength of \(\displaystyle 3.00×10^{−5}T\). What are the direction and magnitude of the torque on the loop?

(b) Does this device have any practical applications as a motor?

82. Repeat Exercise, but with the loop lying flat on the ground with its current circulating counterclockwise (when viewed from above) in a location where the Earth’s field is north, but at an angle \(\displaystyle 45.0º\) below the horizontal and with a strength of \(\displaystyle 6.00×10^{−5}T\).

Solution
(a) \(\displaystyle 0.666 N⋅m\) west
(b) This is not a very significant torque, so practical use would be limited. Also, the current would need to be alternated to make the loop rotate (otherwise it would oscillate).

22.10: Magnetic Force between Two Parallel Conductors

83. (a) The hot and neutral wires supplying DC power to a light-rail commuter train carry 800 A and are separated by 75.0 cm. What is the magnitude and direction of the force between 50.0 m of these wires?

(b) Discuss the practical consequences of this force, if any.

Solution
(a) 8.53 N, repulsive
(b) This force is repulsive and therefore there is never a risk that the two wires will touch and short circuit.

84. The force per meter between the two wires of a jumper cable being used to start a stalled car is 0.225 N/m.

(a) What is the current in the wires, given they are separated by 2.00 cm?

(b) Is the force attractive or repulsive?

85. A 2.50-m segment of wire supplying current to the motor of a submerged submarine carries 1000 A and feels a 4.00-N repulsive force from a parallel wire 5.00 cm away. What is the direction and magnitude of the current in the other wire?

Solution
400 A in the opposite direction

86. The wire carrying 400 A to the motor of a commuter train feels an attractive force of \(\displaystyle 4.00×10^{−3}N/m\) due to a parallel wire carrying 5.00 A to a headlight.

(a) How far apart are the wires?

(b) Are the currents in the same direction?

87. An AC appliance cord has its hot and neutral wires separated by 3.00 mm and carries a 5.00-A current.

(a) What is the average force per meter between the wires in the cord?

(b) What is the maximum force per meter between the wires?

(c) Are the forces attractive or repulsive?

(d) Do appliance cords need any special design features to compensate for these forces?

Solution
(a) \(\displaystyle 1.67×10^{−3}N/m\)
(b) \(\displaystyle 3.33×10^{−3}N/m\)
(c) Repulsive
(d) No, these are very small forces

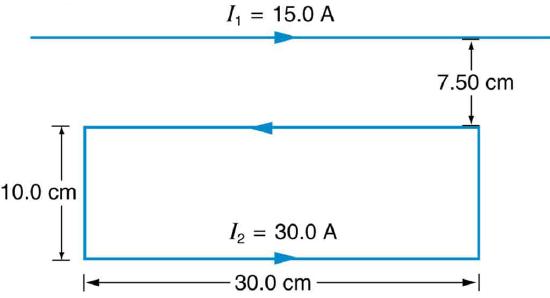
88. Figure shows a long straight wire near a rectangular current loop. What is the direction and magnitude of the total force on the loop?

89. Find the direction and magnitude of the force that each wire experiences in Figure(a) by, using vector addition.

Solution
(a) Top wire: \(\displaystyle 2.65×10^{−4}N/m\) s, \(\displaystyle 10.9º\) to left of up
(b) Lower left wire: \(\displaystyle 3.61×10^{−}4N/m, 13.9º\) down from right
(c) Lower right wire: \(\displaystyle 3.46×10^{−4}N/m, 30.0º\) down from left

90. Find the direction and magnitude of the force that each wire experiences in Figure(b), using vector addition.

22.11 More Applications of Magnetism

91. Indicate whether the magnetic field created in each of the three situations shown in Figure is into or out of the page on the left and right of the current.

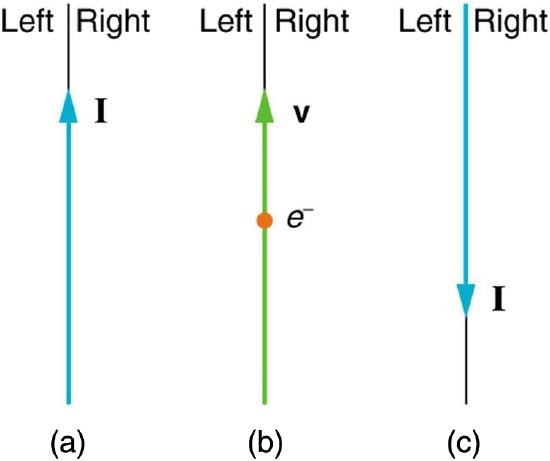
Solution
(a) right-into page, left-out of page
(b) right-out of page, left-into page
(c) right-out of page, left-into page

92. What are the directions of the fields in the center of the loop and coils shown in Figure?

93. What are the directions of the currents in the loop and coils shown in Figure?

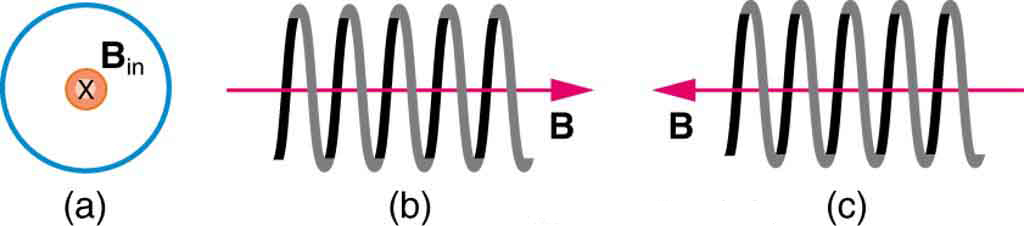
Solution
a) clockwise
(b) clockwise as seen from the left
(c) clockwise as seen from the right

94. To see why an MRI utilizes iron to increase the magnetic field created by a coil, calculate the current needed in a 400-loop-per-meter circular coil 0.660 m in radius to create a 1.20-T field (typical of an MRI instrument) at its center with no iron present. The magnetic field of a proton is approximately like that of a circular current loop \(\displaystyle 0.650×10^{−15}m\) in radius carrying \(\displaystyle 1.05×10^4A\). What is the field at the center of such a loop?

Solution
\(\displaystyle 1.01×10^{13}T\)

95. Inside a motor, 30.0 A passes through a 250-turn circular loop that is 10.0 cm in radius. What is the magnetic field strength created at its center?

96. Nonnuclear submarines use batteries for power when submerged.

(a) Find the magnetic field 50.0 cm from a straight wire carrying 1200 A from the batteries to the drive mechanism of a submarine.

(b) What is the field if the wires to and from the drive mechanism are side by side?

(c) Discuss the effects this could have for a compass on the submarine that is not shielded.

Solution
(a) \(\displaystyle 4.80×10^{−4}T\)
(b) Zero
(c) If the wires are not paired, the field is about 10 times stronger than Earth’s magnetic field and so could severely disrupt the use of a compass.

97. How strong is the magnetic field inside a solenoid with 10,000 turns per meter that carries 20.0 A?

98. What current is needed in the solenoid described in Exercise to produce a magnetic field \(\displaystyle 10^4\) times the Earth’s magnetic field of \(\displaystyle 5.00×10^{−5}T\)?

Solution
39.8 A

99. How far from the starter cable of a car, carrying 150 A, must you be to experience a field less than the Earth’s (\(\displaystyle 5.00×10^{−5}T\))? Assume a long straight wire carries the current. (In practice, the body of your car shields the dashboard compass.)

100. Measurements affect the system being measured, such as the current loop in [link].

(a) Estimate the field the loop creates by calculating the field at the center of a circular loop 20.0 cm in diameter carrying 5.00 A.

(b) What is the smallest field strength this loop can be used to measure, if its field must alter the measured field by less than 0.0100%?

Solution
(a) \(\displaystyle 3.14×10^{−5}T\)
(b) 0.314 T

101. Figure shows a long straight wire just touching a loop carrying a current \(\displaystyle I_1\). Both lie in the same plane.

(a) What direction must the current \(\displaystyle I_2\) in the straight wire have to create a field at the center of the loop in the direction opposite to that created by the loop?

(b) What is the ratio of \(\displaystyle I_1/I_2\) that gives zero field strength at the center of the loop?

(c) What is the direction of the field directly above the loop under this circumstance?

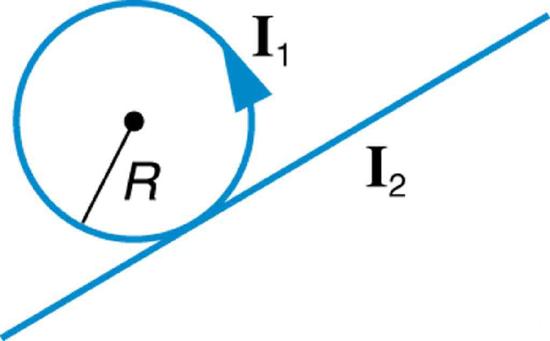
102. Find the magnitude and direction of the magnetic field at the point equidistant from the wires in [link](a), using the rules of vector addition to sum the contributions from each wire.

Solution
\(\displaystyle 7.55×10^{−5}T, 23.4º\)

103. Find the magnitude and direction of the magnetic field at the point equidistant from the wires in [link](b), using the rules of vector addition to sum the contributions from each wire.

104. What current is needed in the top wire in [link](a) to produce a field of zero at the point equidistant from the wires, if the currents in the bottom two wires are both 10.0 A into the page?

Solution
10.0 A

105. Calculate the size of the magnetic field 20 m below a high voltage power line. The line carries 450 MW at a voltage of 300,000 V.

106. Integrated Concepts

(a) A pendulum is set up so that its bob (a thin copper disk) swings between the poles of a permanent magnet as shown in Figure. What is the magnitude and direction of the magnetic force on the bob at the lowest point in its path, if it has a positive \(\displaystyle 0.250 μC\) charge and is released from a height of 30.0 cm above its lowest point? The magnetic field strength is 1.50 T.

(b) What is the acceleration of the bob at the bottom of its swing if its mass is 30.0 grams and it is hung from a flexible string? Be certain to include a free-body diagram as part of your analysis.

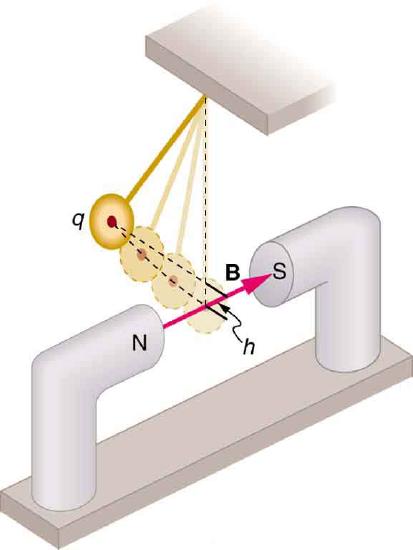
Solution
(a) \(\displaystyle 9.09×10^{−7}N\) upward
(b) \(\displaystyle 3.03×10^{−5}m/s^2\)

107. Integrated Concepts

(a) What voltage will accelerate electrons to a speed of \(\displaystyle 6.00×10^{6}m/s\)?

(b) Find the radius of curvature of the path of a proton accelerated through this potential in a 0.500-T field and compare this with the radius of curvature of an electron accelerated through the same potential.

108. Integrated Concepts

Find the radius of curvature of the path of a 25.0-MeV proton moving perpendicularly to the 1.20-T field of a cyclotron.

Solution
60.2 cm

109. Integrated Concepts

To construct a nonmechanical water meter, a 0.500-T magnetic field is placed across the supply water pipe to a home and the Hall voltage is recorded.

(a) Find the flow rate in liters per second through a 3.00-cm-diameter pipe if the Hall voltage is 60.0 mV.

(b) What would the Hall voltage be for the same flow rate through a 10.0-cm-diameter pipe with the same field applied?

110. Integrated Concepts

(a) Using the values given for an MHD drive in Exercise, and assuming the force is uniformly applied to the fluid, calculate the pressure created in \(\displaystyle N/m^2\).

(b) Is this a significant fraction of an atmosphere?

Solution
(a) \(\displaystyle 1.02×10^3N/m^2\)
(b) Not a significant fraction of an atmosphere

111. Integrated Concepts

(a) Calculate the maximum torque on a 50-turn, 1.50 cm radius circular current loop carrying \(\displaystyle 50 μA\) in a 0.500-T field.

(b) If this coil is to be used in a galvanometer that reads \(\displaystyle 50 μA\) full scale, what force constant spring must be used, if it is attached 1.00 cm from the axis of rotation and is stretched by the \(\displaystyle 60º\) arc moved?

112. Integrated Concepts

A current balance used to define the ampere is designed so that the current through it is constant, as is the distance between wires. Even so, if the wires change length with temperature, the force between them will change. What percent change in force per degree will occur if the wires are copper?

Solution
\(\displaystyle 17.0×10^{−4}%/ºC\)

113. Integrated Concepts

(a) Show that the period of the circular orbit of a charged particle moving perpendicularly to a uniform magnetic field is \(\displaystyle T=2πm/(qB)\).

(b) What is the frequency \(\displaystyle f\)?

(c) What is the angular velocity \(\displaystyle ω\)? Note that these results are independent of the velocity and radius of the orbit and, hence, of the energy of the particle. (Figure.)

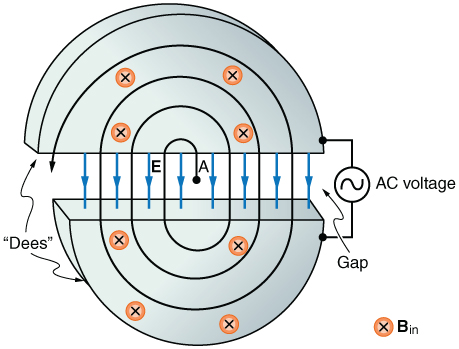
Cyclotrons accelerate charged particles orbiting in a magnetic field by placing an AC voltage on the metal Dees, between which the particles move, so that energy is added twice each orbit. The frequency is constant, since it is independent of the particle energy—the radius of the orbit simply increases with energy until the particles approach the edge and are extracted for various experiments and applications.

114. Integrated Concepts

A cyclotron accelerates charged particles as shown in Figure. Using the results of the previous problem, calculate the frequency of the accelerating voltage needed for a proton in a 1.20-T field.

Solution
18.3 MHz

115. Integrated Concepts

(a) A 0.140-kg baseball, pitched at 40.0 m/s horizontally and perpendicular to the Earth’s horizontal \(\displaystyle 5.00×10^{−5}T\) field, has a 100-nC charge on it. What distance is it deflected from its path by the magnetic force, after traveling 30.0 m horizontally?

(b) Would you suggest this as a secret technique for a pitcher to throw curve balls?

116. Integrated Concepts

(a) What is the direction of the force on a wire carrying a current due east in a location where the Earth’s field is due north? Both are parallel to the ground.

(b) Calculate the force per meter if the wire carries 20.0 A and the field strength is \(\displaystyle 3.00×10^{−5}T\).

(c) What diameter copper wire would have its weight supported by this force?

(d) Calculate the resistance per meter and the voltage per meter needed.

Solution
(a) Straight up
(b) \(\displaystyle 6.00×10^{−4}N/m\)
(c) \(\displaystyle 94.1 μm\)
(d)2.47 Ω/m, 49.4 V/m

117. Integrated Concepts

One long straight wire is to be held directly above another by repulsion between their currents. The lower wire carries 100 A and the wire 7.50 cm above it is 10-gauge (2.588 mm diameter) copper wire.

(a) What current must flow in the upper wire, neglecting the Earth’s field?

(b) What is the smallest current if the Earth’s \(\displaystyle 3.00×10^{−5}T\) field is parallel to the ground and is not neglected?

(c) Is the supported wire in a stable or unstable equilibrium if displaced vertically? If displaced horizontally?

118. Unreasonable Results

(a) Find the charge on a baseball, thrown at 35.0 m/s perpendicular to the Earth’s \(\displaystyle 5.00×10^{−5}T\) field, that experiences a 1.00-N magnetic force.

(b) What is unreasonable about this result?

(c) Which assumption or premise is responsible?

Solution
(a) 571 C
(b) Impossible to have such a large separated charge on such a small object.
(c) The 1.00-N force is much too great to be realistic in the Earth’s field.

119. Unreasonable Results

A charged particle having mass \(\displaystyle 6.64×10^{−27}kg\) (that of a helium atom) moving at \(\displaystyle 8.70×10^5m/s\) perpendicular to a 1.50-T magnetic field travels in a circular path of radius 16.0 mm.

(a) What is the charge of the particle?

(b) What is unreasonable about this result?

(c) Which assumptions are responsible?

120. Unreasonable Results

An inventor wants to generate 120-V power by moving a 1.00-m-long wire perpendicular to the Earth’s \(\displaystyle 5.00×10^{−5}T\) field.

(a) Find the speed with which the wire must move.

(b) What is unreasonable about this result?

(c) Which assumption is responsible?

Solution
(a) \(\displaystyle 2.40×10^6m/s\)
(b) The speed is too high to be practical ≤ 1% speed of light
(c) The assumption that you could reasonably generate such a voltage with a single wire in the Earth’s field is unreasonable

121. Unreasonable Results

Frustrated by the small Hall voltage obtained in blood flow measurements, a medical physicist decides to increase the applied magnetic field strength to get a 0.500-V output for blood moving at 30.0 cm/s in a 1.50-cm-diameter vessel.

(a) What magnetic field strength is needed?

(b) What is unreasonable about this result?

(c) Which premise is responsible?

122. Unreasonable Results

A surveyor 100 m from a long straight 200-kV DC power line suspects that its magnetic field may equal that of the Earth and affect compass readings.

(a) Calculate the current in the wire needed to create a \(\displaystyle 5.00×10^{−5}T\) field at this distance.

(b) What is unreasonable about this result?

(c) Which assumption or premise is responsible?

Solution
(a) 25.0 kA
(b) This current is unreasonably high. It implies a total power delivery in the line of 50.0x10^9 W, which is much too high for standard transmission lines.
(c)100 meters is a long distance to obtain the required field strength. Also coaxial cables are used for transmission lines so that there is virtually no field for DC power lines, because of cancellation from opposing currents. The surveyor’s concerns are not a problem for his magnetic field measurements.

123. Construct Your Own Problem

Consider a mass separator that applies a magnetic field perpendicular to the velocity of ions and separates the ions based on the radius of curvature of their paths in the field. Construct a problem in which you calculate the magnetic field strength needed to separate two ions that differ in mass, but not charge, and have the same initial velocity. Among the things to consider are the types of ions, the velocities they can be given before entering the magnetic field, and a reasonable value for the radius of curvature of the paths they follow. In addition, calculate the separation distance between the ions at the point where they are detected.

124. Construct Your Own Problem

Consider using the torque on a current-carrying coil in a magnetic field to detect relatively small magnetic fields (less than the field of the Earth, for example). Construct a problem in which you calculate the maximum torque on a current-carrying loop in a magnetic field. Among the things to be considered are the size of the coil, the number of loops it has, the current you pass through the coil, and the size of the field you wish to detect. Discuss whether the torque produced is large enough to be effectively measured. Your instructor may also wish for you to consider the effects, if any, of the field produced by the coil on the surroundings that could affect detection of the small field.

Contributors and Attributions

• Paul Peter Urone (Professor Emeritus at California State University, Sacramento) and Roger Hinrichs (State University of New York, College at Oswego) with Contributing Authors: Kim Dirks (University of Auckland) and Manjula Sharma (University of Sydney). This work is licensed by OpenStax University Physics under a Creative Commons Attribution License (by 4.0).