5.11: Total Internal Reflection
( \newcommand{\kernel}{\mathrm{null}\,}\)
Total internal reflection refers to a particular condition resulting in the complete reflection of a wave at the boundary between two media, with no power transmitted into the second region. One way to achieve complete reflection with zero transmission is simply to require the second material to be a perfect conductor. However, total internal reflection is a distinct phenomenon in which neither of the two media are perfect conductors. Total internal reflection has a number of practical applications; notably, it is the enabling principle of fiber optics.
Consider the situation shown in Figure 5.11.1:
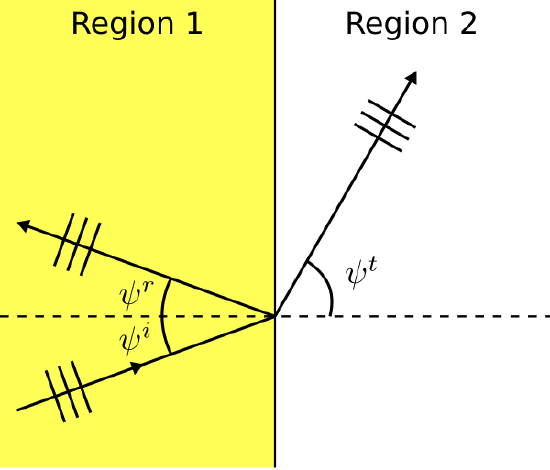 Figure 5.11.1: A uniform plane wave obliquely incident on the planar boundary between two semi-infinite material regions. Here μr1ϵr1>μr2ϵr2, so ψt>ψi (CC BY-SA 4.0 (modified); C. Wang)
Figure 5.11.1: A uniform plane wave obliquely incident on the planar boundary between two semi-infinite material regions. Here μr1ϵr1>μr2ϵr2, so ψt>ψi (CC BY-SA 4.0 (modified); C. Wang)
A uniform plane wave is obliquely incident on the planar boundary between two semi-infinite material regions. In Section 5.8, it is found that
ψr=ψi
i.e., angle of reflection equals angle of incidence. Also, from Snell’s law:
√μr1ϵr1sinψi=√μr2ϵr2sinψt
where “r” in the subscripts indicates the relative (unitless) quantities. The associated formula for ψt explicitly is:
ψt=arcsin(√μr1ϵr1μr2ϵr2sinψi)
From Equation ???, it is apparent that when μr1ϵr1>μr2ϵr2, ψt>ψr; i.e., the transmitted wave appears to bend away from the surface normal, as shown in Figure 5.11.1. In fact, ψt can be as large as π/2 (corresponding to propagation parallel to the boundary) for angles of incidence which are less than π/2. What happens if the angle of incidence is further increased? When calculating ψt using Equation ???, one finds that the argument of the arcsine function becomes greater than 1. Since the possible values of the sine function are between −1 and +1, the arcsine function is undefined. Clearly our analysis is inadequate in this situation.
To make sense of this, let us begin by identifying the threshold angle of incidence ψic at which the trouble begins. From the analysis in the previous paragraph,
√μr1ϵr1μr2ϵr2sinψic=1
therefore,
ψic=arcsin√μr2ϵr2μr1ϵr1
This is known as the critical angle. When ψi<ψic, our existing theory applies. When ψi≥ψic, the situation is, at present, unclear. For non-magnetic materials, Equation ??? simplifies to
ψic=arcsin√ϵr2ϵr1
Now let us examine the behavior of the reflection coefficient. For example, the reflection coefficient for TE component and non-magnetic materials is
ΓTE=cosψi−√ϵr2/ϵr1−sin2ψicosψi+√ϵr2/ϵr1−sin2ψi
From Equation ???, we see that
sin2ψic=ϵr2ϵr1
So, when ψi>ψic, we see that
ϵr2/ϵr1−sin2ψi<0 (ψi>ψic)
and therefore
√ϵr2/ϵr1−sin2ψi=jB (ψi>ψic)
where B is a positive real-valued number. Now we may write Equation ??? as follows:
ΓTE=A−jBA+jB (ψi>ψic)
where A≜cosψi is also a positive real-valued number. Note that the numerator and denominator in Equation ??? have equal magnitude. Therefore, the magnitude of |ΓTE|=1 and
ΓTE=ejζ (ψi>ψic)
where ζ is a real-valued number indicating the phase of ΓTE. In other words,
When the angle of incidence ψi exceeds the critical angle ψic, the magnitude of the reflection coefficient is 1. In this case, all power is reflected, and no power is transmitted into the second medium. This is total internal reflection.
Although we have obtained this result for TE component, the identical conclusion is obtained for TM component as well. This is left as an exercise for the student.
Figure 5.8.2 (Section 5.8) shows a demonstration of refraction of a beam of light incident from air onto a planar boundary with glass. Analysis of that demonstration revealed that the relative permittivity of the glass was ≅2.28. Figure 5.11.2 shows a modification of the demonstration: In this case, a beam of light incident from glass onto a planar boundary with air. Confirm that total internal reflection is the expected result in this demonstration.
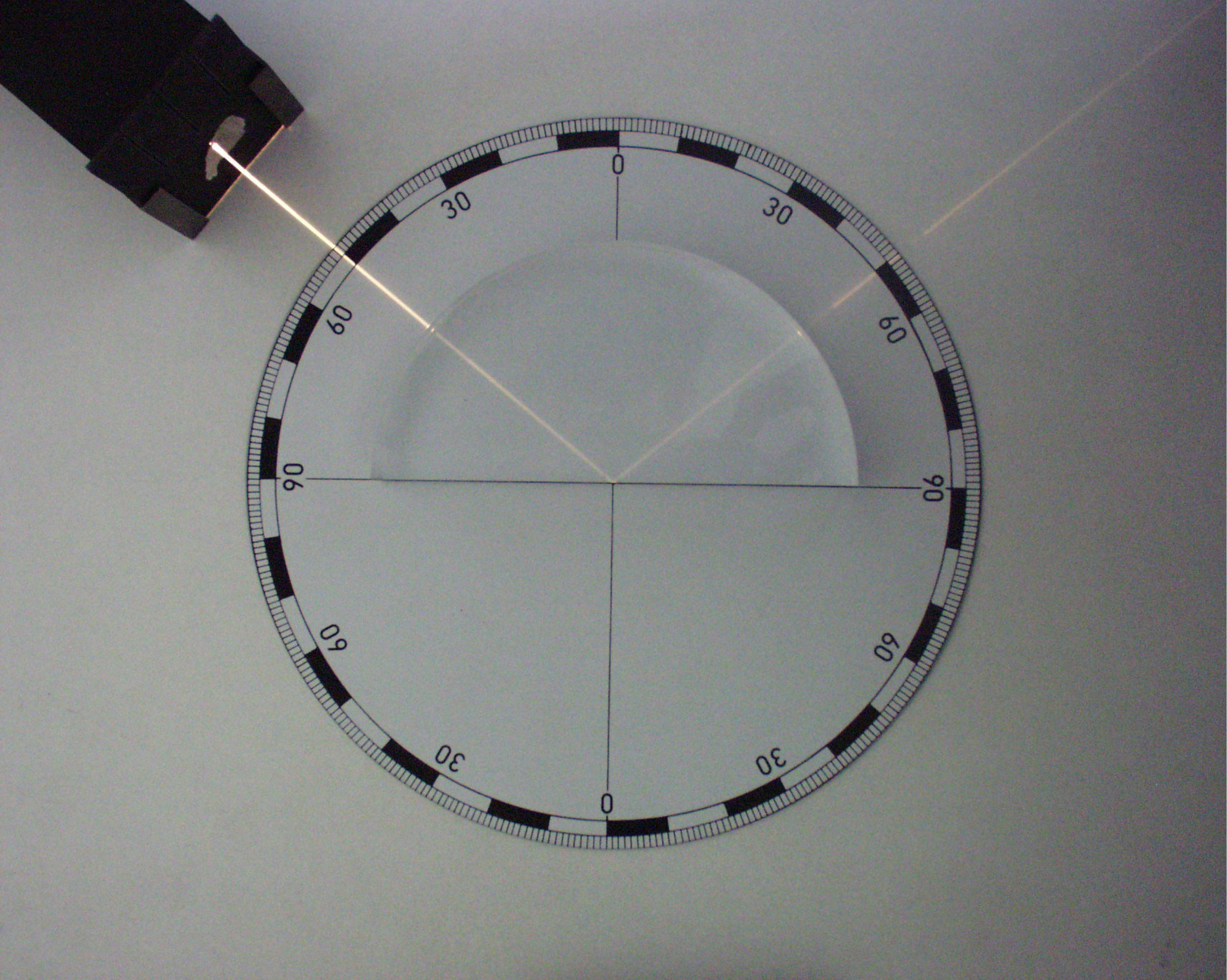 Figure 5.11.2: Total internal reflection of a light wave incident on a planar boundary between glass and air. ( CC BY-SA 3.0; Z. Sándor)
Figure 5.11.2: Total internal reflection of a light wave incident on a planar boundary between glass and air. ( CC BY-SA 3.0; Z. Sándor)
Solution
Assuming the glass is non-magnetic, the critical angle is given by Equation ???. In the present example, ϵr1≅2.28 (glass), ϵr2≅1 (air). Therefore, the critical angle ψic≅41.5∘. The angle of incidence in Figure 5.11.2 is seen to be about ≅50∘, which is greater than the critical angle. Therefore, total internal reflection is expected.
Note also that the phase ζ of the reflection coefficient is precisely zero unless ψi>ψic, at which point it is both non-zero and varies with ψi. This is known as the Goos-Hänchen effect. This leads to the startling phenomenon shown in Figure 5.11.3. In this figure, the Goos-Hänchen phase shift is apparent as a displacement between the point of incidence and the point of reflection.
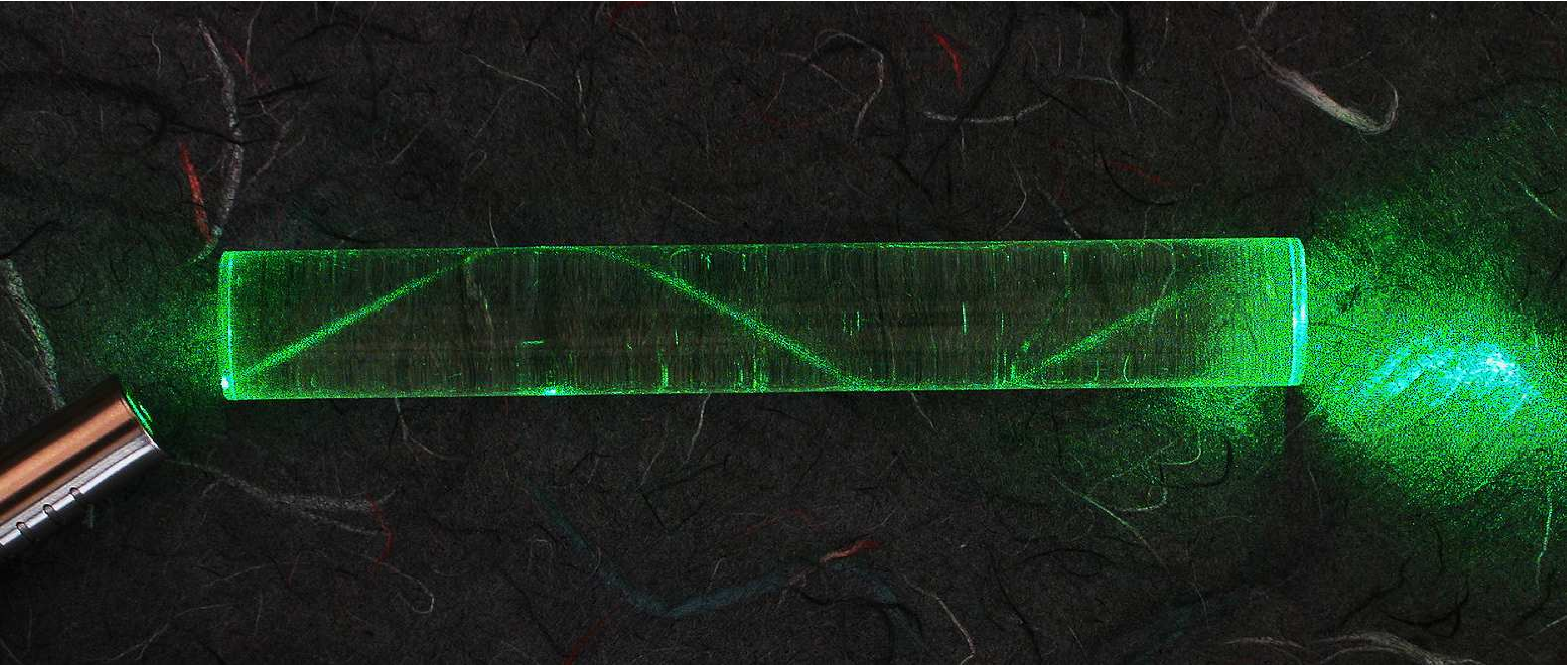 Figure 5.11.3: Laser light in a dielectric rod exhibiting the Goos-Hänchen effect. ( CC BY-SA 3.0; Timwether)
Figure 5.11.3: Laser light in a dielectric rod exhibiting the Goos-Hänchen effect. ( CC BY-SA 3.0; Timwether)
The presence of an imaginary component in the reflection coefficient is odd for two reasons. First, we are not accustomed to seeing a complex-valued reflection coefficient emerge when the wave impedances of the associated media are real-valued. Second, the total reflection of the incident wave seems to contradict the boundary condition that requires the tangential components of the electric and magnetic fields to be continuous across the boundary. That is, how can these components of the fields be continuous across the boundary if no power is transmitted across the boundary? These considerations suggest that there is a field on the opposite side of the boundary, but – somehow – it must have zero power. This is exactly the case, as is explained in Section 5.12.


