6.4: Black Holes (Part 1)

Singularities

A provocative feature of the Schwarzschild metric is that it has elements that blow up at \(r = 0\) and at \(r = 2m\). If this is a description of the sun, for example, then these singularities are of no physical significance, since we only solved the Einstein field equation for the vacuum region outside the sun, whereas \(r = 2m\) would lie about 3 km from the sun’s center. Furthermore, it is possible that one or both of these singularities is nothing more than a spot where our coordinate system misbehaves. This would be known as a coordinate singularity. For example, the metric of ordinary polar coordinates in a Euclidean plane has \(g^{\theta \theta} → \infty\) as \(r → 0\).

One way to test whether a singularity is a coordinate singularity is to calculate a scalar measure of curvature, whose value is independent of the coordinate system. We can take the trace of the Ricci tensor, \(R^a_a\), known as the scalar curvature or Ricci scalar, but since the Ricci tensor is zero, it’s not surprising that that is zero. A different scalar we can construct is the product \(R^{abcd}R_{abcd}\) of the Riemann tensor with itself. This is known as the Kretschmann invariant. The Maxima command lriemann(true) displays the nonvanishing components of R_abcd The component that misbehaves the most severely at r = 0 is R_trrt = \(\frac{2m}{r^{3}}\). Because of this, the Kretschmann invariant blows up like \(r^{−6}\) as \(r → 0\). This shows that the singularity at r = 0 is a real, physical singularity.

The singularity at \(r = 2m\), on the other hand, turns out to be only a coordinate singularity. To prove this, we have to use some technique other than constructing scalar measures of curvature. Even if every such scalar we construct is finite at \(r = 2m\), that doesn’t prove that every such scalar we could construct is also well behaved. We can instead search for some other coordinate system in which to express the solution to the field equations, one in which no such singularity appears. A partially successful change of coordinates for the Schwarzschild metric, found by Eddington in 1924, is (see problem 8):

\[t → t' = t − 2m \ln(r −2m).\]

This makes the covariant metric finite at \(r = 2m\), although the contravariant metric still blows up there. A more complicated change of coordinates that completely eliminates the singularity at \(r = 2m\) was found by Eddington and Finkelstein in 1958, establishing that the singularity was only a coordinate singularity. Thus, if an observer is so unlucky as to fall into a black hole, he will not be subjected to infinite tidal stresses — or infinite anything — at \(r = 2m\). He may not notice anything special at all about his local environment. (Or he may already be dead because the tidal stresses at \(r > 2m\), although finite, were nevertheless great enough to kill him.)

Event Horizon

Even though \(r = 2m\) isn’t a real singularity, interesting things do happen there. For \(r < 2m\), the sign of \(g_{tt}\) becomes negative, while \(g_{rr}\) is positive. In our + − −− signature, this has the following interpretation. For the world-line of a material particle, \(ds^2\) is supposed to be the square of the particle’s proper time, and it must always be positive. If a particle had a constant value of \(r\), for \(r < 2m\), it would have \(ds^2 < 0\), which is impossible.

The timelike and spacelike characters of the \(r\) and \(t\) coordinates have been swapped, so \(r\) acts like a time coordinate.

Thus for an object compact enough that \(r = 2m\) is exterior, \(r = 2m\) is an event horizon: future light cones tip over so far that they do not allow causal relationships to connect with the spacetime outside. In relativity, event horizons do not occur only in the context of black holes; their properties, and some of the implications for black holes, have already been discussed in section 6.1.

The gravitational time dilation in the Schwarzschild field, relative to a clock at infinity, is given by the square root of the \(g_{tt}\) component of the metric. This goes to zero at the event horizon, meaning that, for example, a photon emitted from the event horizon will be infinitely redshifted when it reaches an observer at infinity. This makes sense, because the photon is then undetectable, just as it would be if it had been emitted from inside the event horizon.

Infalling Matter

If matter is falling into a black hole, then due to time dilation an observer at infinity “sees” that matter as slowing down more and more as it approaches the horizon. This has some counterintuitive effects. A radially infalling particle has

\[\frac{d^{2} r}{dt^{2}} > 0\]

once it falls past a certain point, which could be interpreted as a gravitational repulsion. The observer at infinity may also be led to describe the black hole as consisting of an empty, spherical shell of matter that never quite made it through the horizon. If asked what holds the shell up, the observer could say that it is held up by gravitational repulsion.

There is actually nothing wrong with any of this, but one should realize that it is only one possible description in one possible coordinate system. An observer hovering just outside the event horizon sees a completely different picture, with matter falling past at velocities that approach the speed of light as it comes to the event horizon. If an atom emits a photon from the event horizon, the hovering observer sees it as being infinitely red-shifted, but explains the red-shift as a kinematic one rather than a gravitational one.

We can imagine yet a third observer, one who free-falls along with the infalling matter. According to this observer, the gravitational field is always zero, and it takes only a finite time to pass through the event horizon.

If a black hole has formed from the gravitational collapse of a cloud of matter, then some of our observers can say that “right now” the matter is located in a spherical shell at the event horizon, while others can say that it is concentrated at an infinitely dense singularity at the center. Since simultaneity isn’t well defined in relativity, it’s not surprising that they disagree about what’s happening “right now.” Regardless of where they say the matter is, they all agree on the spacetime curvature. In fact, Birkhoff’s theorem tells us that any spherically symmetric vacuum spacetime is Schwarzschild in form, so it doesn’t matter where we say the matter is, as long as it’s distributed in a spherically symmetric way and surrounded by vacuum.

A particularly nice way of summarizing and understanding these issues is with the use of a Penrose diagram, as discussed in section 7.3.

Expected Formation

Einstein and Schwarzschild did not believe, however, that any of these features of the Schwarzschild metric were more than a mathematical curiosity, and the term “black hole” was not invented until the 1967, by John Wheeler. There is quite a bit of evidence these days that our universe does contain objects that have undergone complete gravitational collapse, in the sense that their mass M is contained within a radius \(r \lesssim M\) (in geometrized units). These objects are probably black holes, although doubts have been raised recently as to whether they are in fact other objects such as naked singularities.¹⁰ Supposing that black holes do exist, there is also the question of what sizes they come in.

We might expect naively that since gravity is an attractive force, there would be a tendency for any primordial cloud of gas or dust to spontaneously collapse into a black hole. But clouds of less than about 0.1M_\(\odot\) (0.1 solar masses) form planets, which achieve a permanent equilibrium between gravity and internal pressure. Heavier objects initiate nuclear fusion, but those with masses above about 100M_\(\odot\) are immediately torn apart by their own solar winds. In the range from 0.1 to 100M_\(\odot\), stars form. As discussed in section 4.4, those with masses greater than about a few M_\(\odot\) are expected to form black holes when they die. We therefore expect, on theoretical grounds, that the universe should contain black holes with masses ranging from a few solar masses to a few tens of solar masses.

Observational Evidence

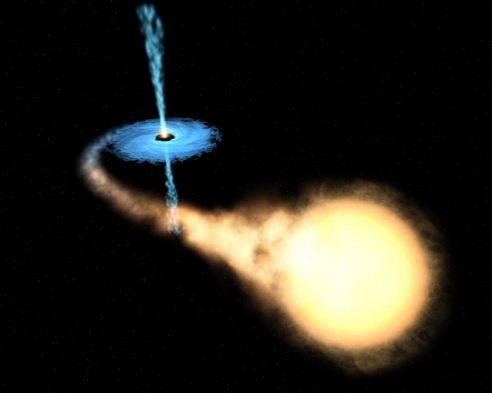
A black hole is expected to be a very compact object, with a strong gravitational field, that does not emit any of its own light. A bare, isolated black hole would be difficult to detect, except perhaps via its lensing of light rays that happen to pass by it. But if a black hole occurs in a binary star system, it is possible for mass to be transferred onto the black hole from its companion, if the companion’s evolution causes it to expand into a giant and intrude upon the black hole’s gravity well. The infalling gas would then get hot and emit radiation before disappearing behind the event horizon (Figure \(\PageIndex{1}\)). The object known as Cygnus X-1 is the best-studied example. This X-ray-emitting object was discovered by a rocket-based experiment in 1964. It is part of a double-star system, the other member being a blue supergiant. They orbit their common center of mass with a period of 5.6 days. The orbit is nearly circular, and has a semimajor axis of about 0.2 times the distance from the earth to the sun. Applying Kepler’s law of periods to these data constrains the sum of the masses, and knowledge of stellar structure fixes the mass of the supergiant. The result is that the mass of Cygnus X-1 is greater than about 10 solar masses, and this is confirmed by multiple methods. Since this is far above the Tolman-Oppenheimer-Volkoff limit, Cygnus X-1 is believed to be a black hole, and its X-ray emissions are interpreted as the radiation from the disk of superheated material accreting onto it from its companion. It is believed to have more than 90% of the maximum possible spin for a black hole of its mass.¹¹

Figure \(\PageIndex{1}\): A black hole accretes matter from a companion star. (Public Domain; NASA).

Around the turn of the 21st century, new evidence was found for the prevalence of supermassive black holes near the centers of nearly all galaxies, including our own. Near our galaxy’s center is an object called Sagittarius A*, detected because nearby stars orbit around it. The orbital data show that Sagittarius A* has a mass of about four million solar masses, confined within a sphere with a radius less than 2.2 × 10⁷ km. There is no known astrophysical model that could prevent the collapse of such a compact object into a black hole, nor is there any plausible model that would allow this much mass to exist in equilibrium in such a small space, without emitting enough light to be observable.

The existence of supermassive black holes is surprising. Gas clouds with masses greater than about 100 solar masses cannot normally form stable stars, so supermassive black holes cannot be the end-point of the evolution of heavy stars. Mergers of multiple stars to form more massive objects are generally statistically unlikely, since a star is such a small target in relation to the distance between the stars. Once astronomers were confronted with the empirical fact of their existence, a variety of mechanisms was proposed for their formation. Little is known about which of these mechanisms is correct, although the existence of quasars in the early universe is interpreted as evidence that mass accreted rapidly onto supermassive black holes in the early stages of the evolution of the galaxies. As of 2016, an explanation getting a lot of attention is that in the early universe, there was a brief period in which the ambient conditions allowed the creation of supermassive black holes by direct collapse.¹²

A skeptic could object that although Cygnus X-1 and Sagittarius A* are more compact than is believed possible for a neutron star, this does not necessarily prove that they are black holes. Indeed, speculative theories have been proposed in which exotic objects could exist that are intermediate in compactness between black holes and neutron stars. These hypothetical creatures have names like black stars, gravastars, quark stars, boson stars, Q-balls, and electroweak stars. Although there is no evidence that these theories are right or that these objects exist, we are faced with the question of how to determine whether a given object is really a black hole or one of these other species. The defining characteristic of a black hole is that it has an event horizon rather than a physical surface. We currently have two ways of probing the structure of these stars at the radii where general relativity predicts the existence of an event horizon.

If an object is not a black hole, then by conservation of energy any matter that falls onto it must release its gravitational potential energy when it hits that surface. Cygnus X-1 has a copious supply of matter falling onto it from its supergiant companion, and Sagittarius A* likewise accretes a huge amount of gas from the stellar wind of nearby stars. By analyzing millimeter and infrared very-long-baseline-interferometry observations, Broderick, Loeb, and Narayan¹³ have shown that if Sagittarius A* had a surface, then the luminosity of this surface must be less than 0.3% of the luminosity of the accretion disk. But this is not physically possible, because there are fundamental limits on the efficiency with which the gas can radiate away its energy before hitting the surface. We can therefore conclude that Sagittarius A* must have an event horizon. Its event horizon may be imaged directly in the near future.¹⁴

A second approach is through the observation of gravitational waves. As discussed in more detail in ch. 9, 2016 saw the first direct observation of gravitational waves. The waveform that was detected (Figure 9.2.2) fits very well with the predictions of general relativity for the merger of two black holes. It seems very unlikely that a waveform with this time-scale and characteristic shape could have been produced unless general relativity’s description of black holes is correct in detail.

Singularities and Cosmic Censorship

Informal Ideas

Since we observe that black holes really do exist, maybe we should take the singularity at r = 0 seriously. Physically, it says that the mass density and tidal forces blow up to infinity there.

Generally when a physical theory says that observable quantities blow up to infinity at a particular point, it means that the theory has reached the point at which it can no longer make physical predictions. For instance, Maxwell’s theory of electromagnetism predicts that the electric field blows up like r⁻² near a point charge, and this implies that infinite energy is stored in the field within a finite radius around the charge. Physically, this can’t be right, because we know it only takes 511 keV of energy to create an electron out of nothing, e.g., in nuclear beta decay. The paradox is resolved by quantum electrodynamics, which modifies the description of the vacuum around the electron to include a sea of virtual particles popping into and out of existence.

In the case of a black hole singularity, it is possible that quantum mechanical effects at the Planck scale prevent the formation of a singularity. Unfortunately, we are unlikely to find any empirical evidence about this, since black holes always seem to come clothed in event horizons, so we outside observers cannot extract any data about the singularity inside. Even if we take a suicidal trip into a black hole, we get no data about the singularity, because the singularity in the Schwarzschild metric is spacelike, not timelike, and therefore it always lies in our future light cone, never in our past.

In a way, the inaccessibility of singularities is a good thing. If a singularity exists, it is a point at which all the known laws of physics break down, and physicists therefore have no way of predicting anything about its behavior. There is likewise no great crisis for physics due to the Big Bang singularity or the Big Crunch singularity that occurs in some cosmologies in which the universe recollapses; we have no reasonable expectation of being able to make and test predictions or retrodictions that extend beyond the beginning or end of the universe.

What would be a crushing blow to the enterprise of physics would be a singularity that could sit on someone’s desk. As John Earman of the University of Pittsburgh puts it, anything could pop out of such a “naked” singularity (defined formally later), including green slime or your lost socks.

Penrose’s cosmic censorship conjecture states that the laws of physics prevent the formation of naked singularities from nonsingular and generic initial conditions. “Generic” is a necessary addition to Penrose’s original 1969 formulation, since Choptuik showed in 1993 that certain perfectly fine-tuned initial conditions allowed collapse to a naked singularity.¹⁵ As of 2017, evidence is accumulating that cosmic censorship is false. This is discussed at greater length later.

Formal Definitions

The remainder of this subsection provides a more formal exposition of the definitions relating to singularities. It can be skipped without loss of continuity.

The reason we care about singularities is that they indicate an incompleteness of the theory, and the theory’s inability to make predictions. One of the simplest things we could ask any theory to do would be to predict the trajectories of test particles. For example, Maxwell’s equations correctly predict the motion of an electron in a uniform magnetic field, but they fail to predict the motion of an electron that collides head-on with a positron. It might have been natural for someone in Maxwell’s era (assuming they were informed about the existence of positrons and told to assume that both particles were pointlike) to guess that the two particles would scatter through one another at \(\theta\) = 0, their velocities momentarily becoming infinite. But it would have been equally natural for this person to refuse to make a prediction.

Similarly, if a particle hits a black hole singularity, we should not expect general relativity to make a definite prediction. It doesn’t, because the geodesic equation breaks down.

We would therefore like to define a singularity as a situation in which the geodesics of test particles can’t be extended indefinitely. But what does “indefinitely” mean? If the test particle is a photon, then the metric length of its world-line is zero. We get around this by defining length in terms of an affine parameter.

Definition

A spacetime is said to be geodesically incomplete if there exist timelike or lightlike geodesics that cannot be extended beyond some finite affine parameter into the past or future.

This is also a pretty good working definition of what we mean when we say that a spacetime contains a singularity, although it may not be optimal for all purposes.¹⁶ The Schwarzschild spacetime has a singularity at r = 0, but not at the event horizon, since geodesics continue smoothly past the event horizon. Cosmological spacetimes contain a Big Bang singularity which prevents geodesics from being extended beyond a certain point in the past.

Actual singularities involving geodesic incompleteness are to be distinguished from coordinate singularities, which are not really singularities at all. In the Schwarzschild spacetime, as described in Schwarzschild’s original coordinates, some components of the metric blow up at the event horizon, but this is not an actual singularity. This coordinate system can be replaced with a different one in which the metric is well behaved.

Example 1: A harmless blow-up

Let’s define coordinates (t, y) in the region of spacetime where you’re sitting and reading this book. Let (0, 0) be your current time and position, and for convenience let this be an inertial frame (so that your motion is not geodesic). The Riemann tensor, expressed in these coordinates, has a component R_tyyt = \(\frac{2Gm}{r^{3}}\), where m and r are the mass and radius of the earth. This has the finite value of 1.5 × 10⁻⁶ s⁻², which expresses the strength of a tidal effect near the earth’s surface.

Now define a new coordinate u = y³. Applying the tensor transformation law, we have

\[R_{tuut} = R_{tyyt} \left(\dfrac{\partial y}{\partial u}\right)^{2},\]

which is infinite at y = 0. This example demonstrates that we cannot test for a singularity by looking for a blow-up of the components of a curvature tensor at certain coordinates or as the coordinates approach some limit.

There are two types of singularities: curvature singularities and non-curvature singularities.

The big bang and black hole singularities are examples of curvature singularities, which can often be recognized because there are scalar measures of curvature such as R^abcdR_abcd, known as the Kretschmann invariant, that blow up. These indicate that tidal forces blow up to infinity, and would destroy any observer.

The reason curvature scalars are useful as tests for a curvature singularity is that since they’re scalars, they can’t diverge in one coordinate system but stay finite in another (cf. example 1). A sufficient condition for a singularity to be a curvature singularity is if timelike or lightlike geodesics can only be extended to some finite affine parameter, and some curvature scalar (not necessarily every such scalar) approaches infinity as we approach this value of the affine parameter.

But we should not expect this to be a necessary condition for a curvature singularity. Example 2 below shows that the most commonly occurring curvature scalars may not be enough to catch the presence of a singularity. This is not too surprising, since curvature scalars do not suffice to tell us everything there is to know about the curvature of a spacetime (example 3).

Example 2: Incompleteness with finite curvature scalars

Consider the 1+1-dimensional spacetime described by the metric

\[\begin{split} ds^{2} &= A(dt^{2} - dx^{2}) \\ A &= \frac{1}{1 + e^{t}}, \end{split}\]

with \(− \infty < x < \infty\) and \(− \infty < t < \infty\). For large negative t it is indistinguishable from Minkowski space. The following Maxima code computes its Riemann tensor and the scalar curvature R and the Kretchmann invariant K.

The results for the two curvature scalars are

\[R = (1 + e^{-t})^{-1}\]

and

\[K = (1 + e^{-t})^{-2},\]

both of which are finite everywhere; they go from 0 at large negative times to 1 at large positive times.

From these results we would not imagine that there was any singularity present, but blow-ups of curvature scalars are only a sufficient condition for geodesic incompleteness, not a necessary one. Consider the timelike curve x = 0, which by symmetry is a geodesic. If we integrate the proper time along this geodesic, we get a finite limit as t → \(\infty\). Since proper time qualifies as an affine parameter, this geodesic is incomplete.

But it is not so obvious that this spacetime is “really” singular. It is possible that we could smoothly extend it beyond t = \(+ \infty\). If so, then the singularity at t = \(+ \infty\) would be a kind of fake singularity, of the type that we could obtain simply by chopping off the part of Minkowski space with t ≥ 0.

Example 3: Vanishing curvature scalars

We remarked above that curvature scalars do not in general suffice to tell us everything about the curvature of a spacetime. In fact, there is an entire class of curved spacetimes such that every curvature invariant vanishes everywhere. Schmidt¹⁷ gives the example

\[ds^{2} = du dv - a^{2} (u) dw^{2},\]

where a is an arbitrary nonlinear function. The eigenvalues of this metric are 1, −1, and −a², so its signature is + − −, i.e., this is general relativity in 2 + 1 dimensions. A computation shows that the space is not flat, since, e.g., \(R_{uu} = − \frac{a''}{a}\). The u and v directions are lightlike, so this metric represents a wavelike disturbance traveling at the speed of light. (Since the Ricci tensor doesn’t vanish, this isn’t a vacuum solution, and we don’t have a gravitational wave in vacuum. Such waves, as described in ch. 9, are transverse and can only exist in 3 + 1 or more dimensions.)

The lightlike character of u and v motivates us to consider coordinate transformations of the form (u, v) → (uD, \(\frac{v}{D}\)), because in the case a = 0, which is flat, this would be a Lorentz boost with a Doppler shift factor D. In the case where D approaches zero, we are chasing the wave at a velocity approaching c, so the wave Doppler-shifts to undetectability. All components of the Riemann tensor, as well as their derivatives, approach zero.

Now consider any curvature scalar I that is expressible as a continuous function of the Riemann tensor and its derivatives. By continuity, I approaches zero as D → 0. But curvature scalars are scalars, so they are invariant under coordinate transformations. It therefore follows that I = 0 identically, regardless of the value of D. Thus we have a spacetime that, although curved, has no nonvanishing curvature scalars anywhere.

---

¹⁷ “Why do all the curvature invariants of a gravitational wave vanish?,” arxiv.org/abs/gr-qc/9404037

References

¹⁰ See sec. 6.3, and, e.g., Joshi et al., arxiv.org/abs/1304.7331.

¹¹ Gou et al., “The Extreme Spin of the Black Hole in Cygnus X-1,” http://arxiv.org/abs/1106.3690

¹² See, e.g., http://arxiv.org/abs/1402.5675

¹³ arxiv.org/abs/0903.1105

¹⁴ arxiv.org/abs/0906.4040

¹⁵ Phys. Rev. Lett. 70, p. 9

¹⁶ Geroch, ”What is a singularity in general relativity?,” Ann Phys 48 (1968) 526.