3.3: Mechanical Advantage and Power

Reconciling Work with Mechanical Advantage

Back when we first talked about pulleys, we said that the block-and-tackle device was useful for lifting heavy objects. Figure 3.3.1 shows two blocks lifted the same distance by pulling on a rope in a pulley system. We know that it requires less force to lift the same mass for the case on the left than the case on the right, but now let's compare the amount of work done by the pull force in the two cases. In both cases, the block is raised the same distance, and in both cases it starts and ends at rest.

Figure 3.3.1 – Work Done with Pulley Systems

The pull force acts downward on the end off the rope, and the direction the end of the rope moves is downward, so there is positive work done in both cases. With identical blocks, the force required to be applied to the rope for the left case is half as great as the force required to lift the block in the right case. However, in order to lift the block the same distance from where it started, the rope must be pulled twice as far in the right case than in the left case, thanks to the pulley ratio constraint. With half the force acting over twice the distance, the amount of work done is the same. The same result comes from more pulleys, since the pulley constraint ratio is the same as the mechanical advantage in every case.

So it appears that the block & tackle (and simple machines more generally) trade effort (force) for displacement, such that the amount of work done remains the same. Let's see if we see a similar result for the case of the inclined plane.

Figure 3.3.2 – Work Done with an Inclined Plane

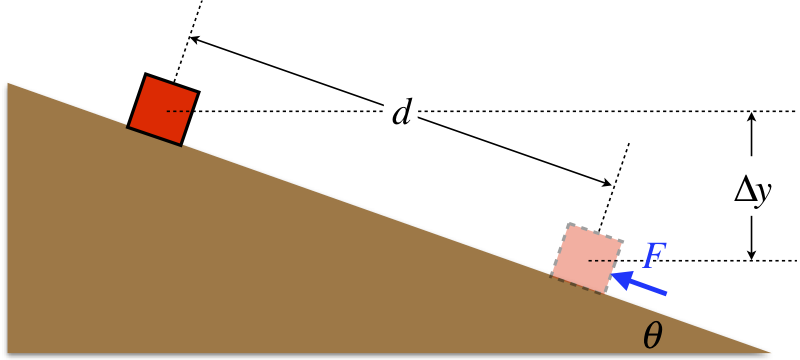
Let's assume that the inclined plane shown in Figure 3.3.2 is frictionless, that the force applied to the block is parallel to the plane, and that it is just enough so that the block moves at a constant speed up the plane. A FBD (without the irrelevant normal force which acts perpendicular to the motion) looks like:

Figure 3.3.3 – Partial FBD of Block on Plane

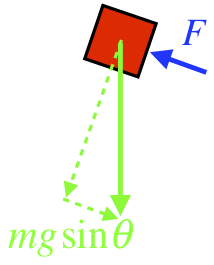
For the block to stay at a constant speed, the force up the plane must equal the force down the plane, which means:

\[ F = mg \sin \theta \]

So the force required is reduced by the factor \(\sin \theta \) compared to lifting the block straight up. But from trigonometry, the distance the block must be pushed is:

\[ d = \frac{\Delta y}{ \sin \theta } \]

When we multiply the force by the distance to get the work done, we get:

\[ W = Fd = \left( mg \sin \theta \right)\left( \frac{\Delta y}{ \sin \theta } \right) = mg \Delta y \]

This is the amount of work required to lift the block straight up at a constant speed, so once again the simple machine trades extra distance for less force to get the same work. How do we know that \( mg \Delta y \) is the work required to lift the block up at a constant speed? We know that to lift it at a constant speed, the net vertical force needs to be zero, which means the upward force needs to balance the gravity force. Multiplying that force by the distance it is raised gives the straight-lift work of ( \(\Delta y \)).

Power

We take a moment now to introduce yet another physics word whose common usage in English is very different from its meaning in physics.

Definition: Power

Power is the rate at which work is performed.

Note that just like we can talk about the work done by an individual force or a collection of forces, we can also talk about the power "delivered" to a system by one or more forces. For example, if a car is moving at a constant speed on level ground, its kinetic energy is not changing over time, so no total work is being done on it. If no work is done on it over time, there is no power being delivered to it. But clearly the engine of the car is doing something. So it is useful to break up the power delivered by separate sources if we want to isolate the rate at which the engine is doing work, without worrying about the rate at which air resistance and friction are are doing negative work on the car to bring the total to zero.

Mathematically, we therefore have for the power delivered by a given force is:

\[ P \equiv \dfrac{dW}{dt} \]

Since the units of work is Joules, the units of power is Joules per second, which we rename as: watts (\(W\)).

One nice shortcut for power involves the force doing the work and the velocity of the object on which the work is being performed:

\[ dW = \overrightarrow F \cdot \overrightarrow{dl} \;\;\; \Rightarrow \;\;\; P = \dfrac{dW}{dt} = \dfrac{\overrightarrow F \cdot \overrightarrow{dl}}{dt} = \overrightarrow F \cdot \dfrac{\overrightarrow {dl}}{dt} = \overrightarrow F \cdot \overrightarrow v \]

Note that this is the power delivered to the moving object (i.e. the rate at which energy is added to or taken away from the object) at the instant that the force and velocity are the vectors given above. If this is integrated from a starting time to a final time, the result is the total work done over that time span by the force.

Example \(\PageIndex{1}\)

An object with a mass of \(0.400kg\) experiences a net force of \(12.0N\) that causes it to speed up as it moves in a circular path of radius \(0.800m\). Find the power delivered to the object at the moment that its speed reaches \(3.00 \dfrac{m}{s}\).

Solution

The net force consists of two perpendicular components: One that speeds up the object, and one that keeps it moving in a circle. We start by calculating the component of the net force that is causing the centripetal acceleration:

\[ F_{\bot} = m \dfrac{v^2}{R} = 4.50N \nonumber \]

With this component of the force and the magnitude of the total force, we can compute the component of the force tangent to the circle:

\[ F_{\parallel} = \sqrt{ F^2 - F_{\bot}^2} = 11.1N \nonumber \]

This is the part of the net force that is parallel to the velocity, so the dot product of the net force and the velocity is just this number multiplied by the speed:

\[ P = \overrightarrow F_{net} \cdot \overrightarrow v = F_{\parallel} \; v = \boxed{33.3W} \nonumber \]