8.7: Chapter Checklist

You should now be able to:

1. Construct traveling wave modes of an infinite system with translation invariance;
2. Decompose a traveling wave into a pair of standing waves, and a standing wave into a pair of traveling waves “moving” in opposite directions;
3. Solve forced oscillation problems with traveling wave solutions and compute the forces acting on the system.
4. Compute the power and average power required to produce a wave, and define and calculate the impedance;
5. Analyze translation invariant systems with damping;
6. Understand the physical origins of high and low frequency cut-offs and be able to analyze the behavior of systems driven above and below the cut-off frequencies.

Problems

8.1. An infinite string with tension $T$ and linear mass density $\rho$ is stretched along the $x$ axis. A force is applied in the $y$ direction at $x = 0$ so as to cause the string at $x = 0$ to oscillate in the $y$ direction with displacement $$A(t)=D \cos \omega t .$$

This produces two traveling waves moving away from $x = 0$ in the $\pm x$ directions.

1. Find the force applied at $x = 0$.
2. Find the average power supplied by the force.

8.2. For air at standard temperature and pressure, the pressure is $1.01 \times 10^{6} \mathrm{dyne} / \mathrm{cm}^{2}$, the density is $1.29 \times 10^{3} \mathrm{gr} / \mathrm{cm}^{3}$. Use these to find the displacement amplitude for sound waves with a frequency of $440 \mathrm {cycles} / \mathrm{sec}$ (Hertz) carrying a power per unit area of $10^{-3} \mathrm {watts} / \mathrm{cm}^{2}$.

8.3. Consider the following circuit:

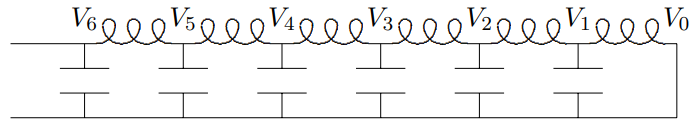
All the capacitors have the same capacitance, $C \approx 0.00667 \mu F,$, and all the inductors have the same inductance, $L \approx 150 \mu H$ and the same resistance, $R \approx 15 \Omega$ (this is the same problem as (5.4), but with nonzero resistance). The wire at the bottom is grounded so that $V_{0} = 0$. This circuit is an electrical analog of the translation invariant systems of coupled mechanical oscillators that we have discussed in this chapter.

1. Show that the dispersion relation for this system is $$\omega^{2}+i \omega \frac{R}{L}=\frac{2}{L C}(1-\cos k a) .$$
   When you apply a harmonically oscillating signal from a signal generator through a coaxial cable to $V_{6}$, different oscillating voltages will be induced along the line. That is if $$V_{6}(t)=V \cos \omega t ,$$
   then $V_{j}(t)$ has the form $$V_{j}(t)=A_{j} \cos \omega t+B_{j} \sin \omega t .$$
2. Find $A_{1}$ and $B_{1}$ and $\left|A_{1}+i B_{1}\right|$ and graph each of them versus $\omega$ from $\omega = 0$ to $2 / \sqrt{L C}$. Never mind simplifying complicated expressions, so long as you can graph them. How many of the resonances can you identify in each of the graphs? Hint: Use the trigonometric identity of problem (1.2e), $$\sin 6 x=\sin x\left(32 \cos ^{5} x-32 \cos ^{3} x+6 \cos x\right)$$
   to express $A_{1} + iB_{1}$ in terms of $\cos k a$. Note that this identity is true even if $x$ is a complex number. Then use the dispersion relation to express $\cos k a$ in terms of $\omega$. Find $A_{1}$ and $B_{1}$ by taking the real and imaginary parts of $A_{1} + iB_{1}$. Finally, program a computer to construct the graphs.⁶
3. Find the positions of the resonances directly using the arguments of chapter 5, and show that they are where you expect them.

___________________
⁶This hint dates from the days before Mathematica was generally available. You may choose to to the problem differently, and that is OK as long as you explain clearly what you are doing and understand it!